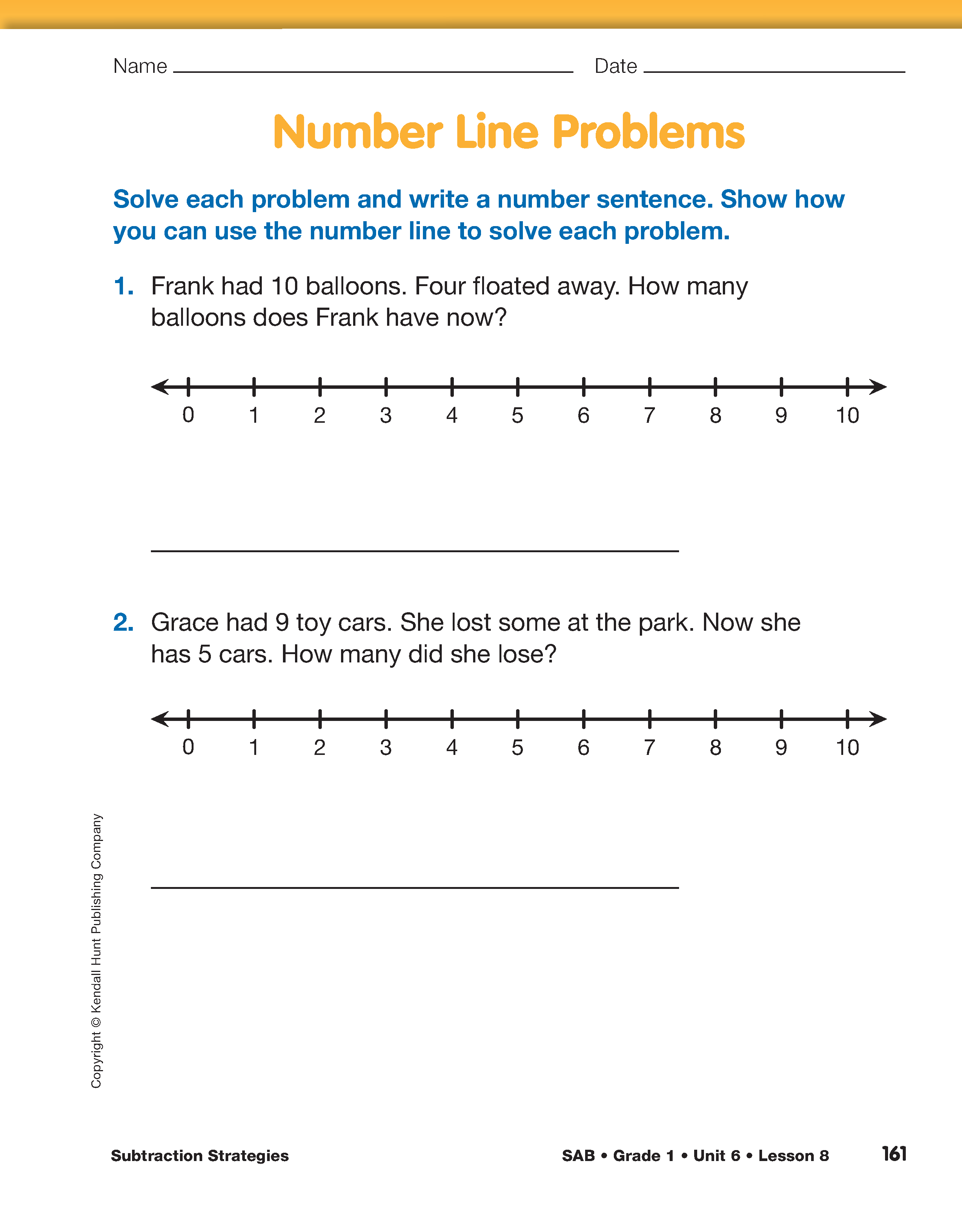
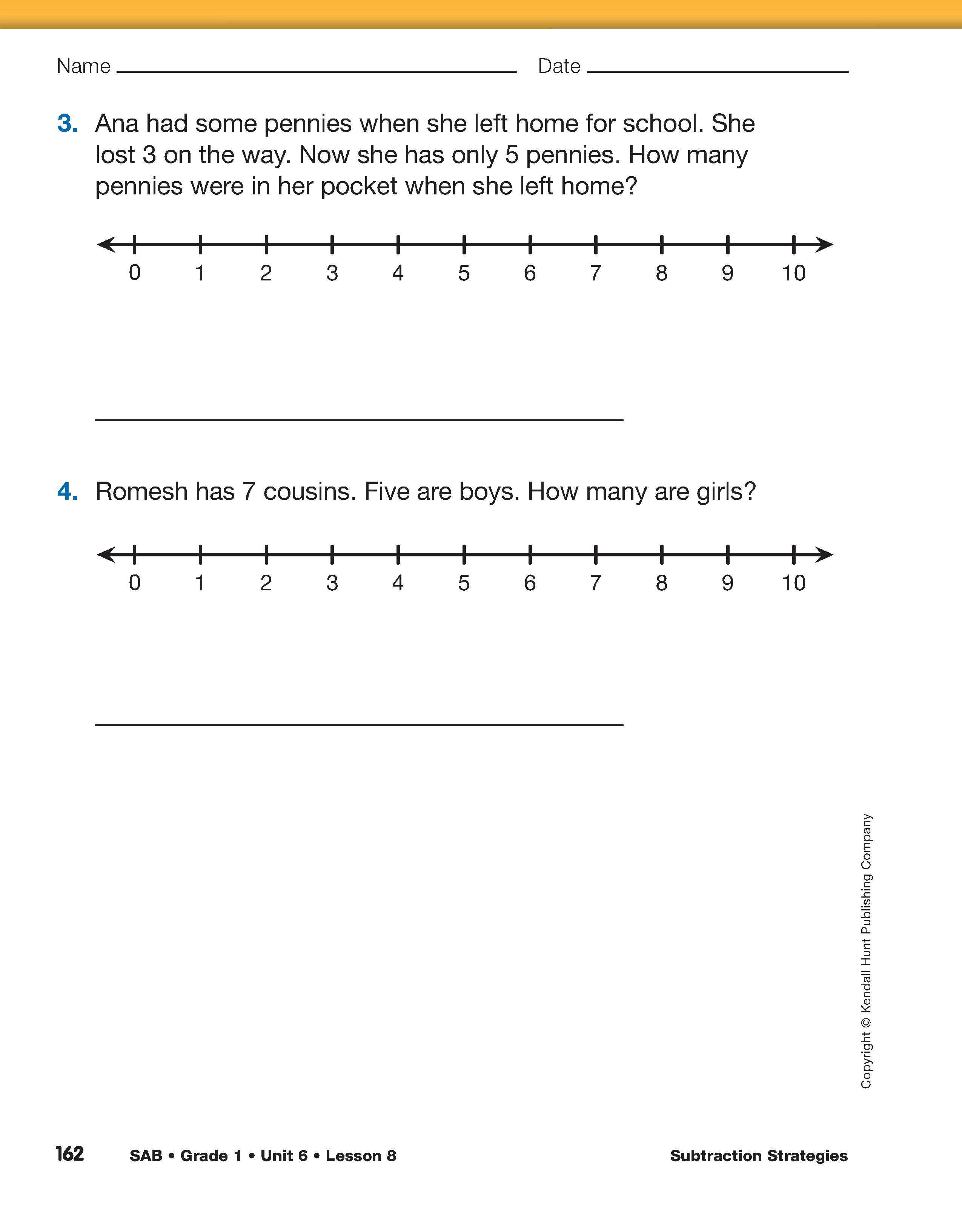
Subtraction Strategies

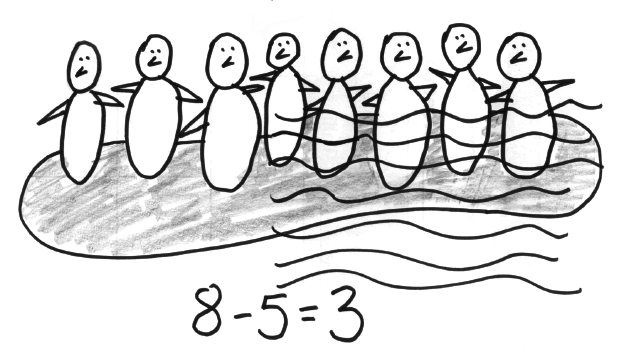
Ask students to draw a simple picture to show what is happening in the problem. Monitor the work and look for evidence that each student understands the whole and the known part and further understands that the action is take away or separate. For example, an understanding of the whole could be shown by a drawing of exactly 8 total objects (penguins). Similarly, an understanding of the known part could be shown by separating out five of those objects or distinguishing two groups in some other way such as drawing a circle around them or crossing them out. See Figure 7.

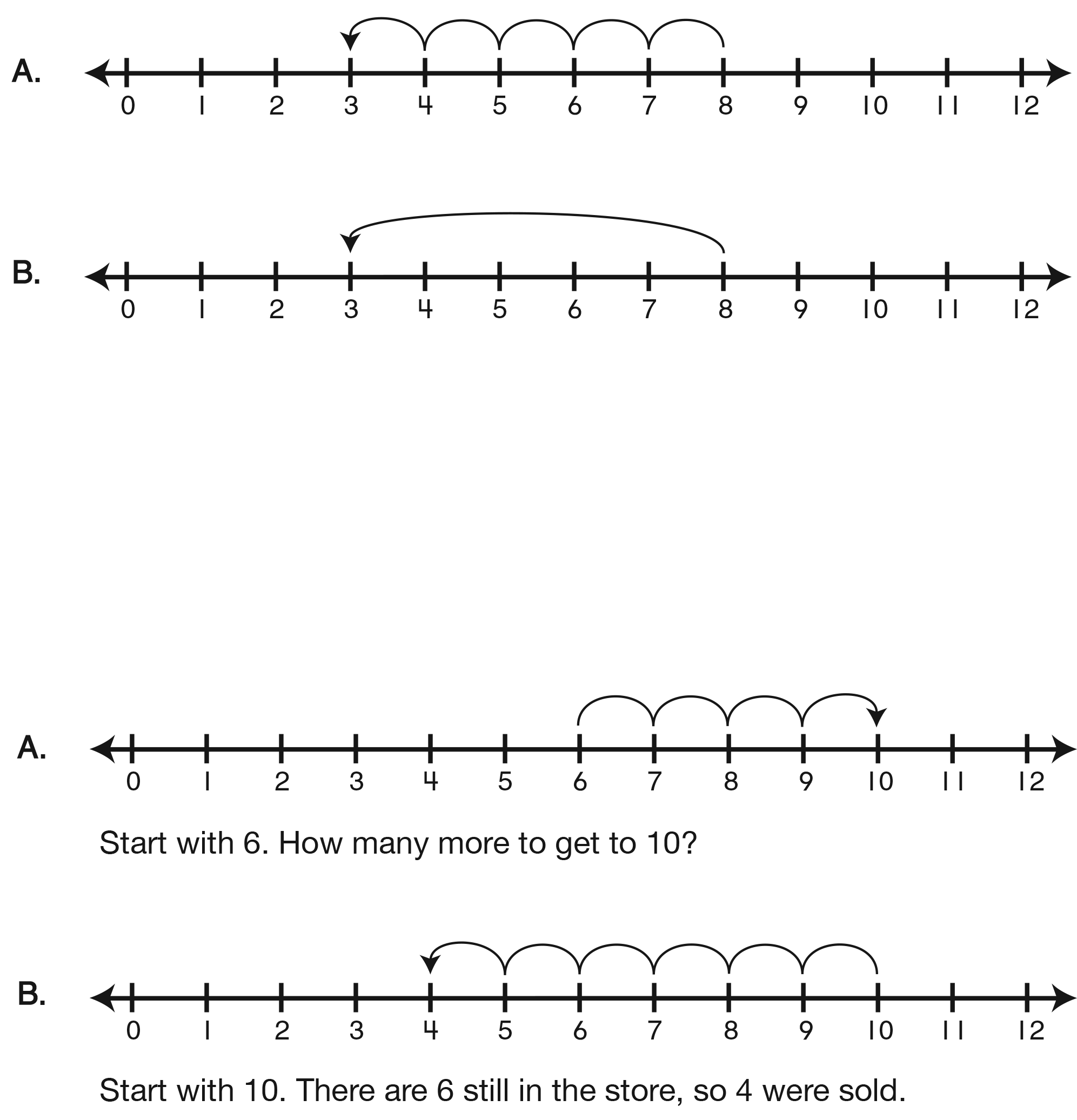
Use the question to start a discussion of how to use a number line to solve subtraction problems. Have a student demonstrate how to solve the problem on the class number line. See Content Note. Possible strategies are shown in Figure 8.

Ask a student to draw a picture on the board of what is happening in the picture. He or she might show 10 stuffed polar bears, and then cross out some until 6 are left. The four crossed out shows the answer to the problem.

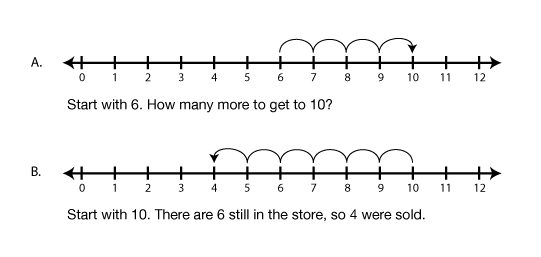
Refer back to number lines. Since this problem is not a take-away situation, the strategies will likely be somewhat different. Possible representations on the number line are shown in Figure 9. If students think of the problem as a missing addend problem (6 polar bears in the store + bears sold = 10 polar bears total), they may represent the situation as shown in first number line (A). The second number line (B) shows another possible interpretation. Encourage students to develop representations that make sense to them. Then ask them to explain their reasoning to the class as a check on the logic and clarity of their representations and explanations.

Ask students to work in pairs to use number lines to solve the problems on the Number Line Problems pages in the Student Activity Book. These problems include a variety of problem types and can be represented on the number line in different ways. Since the problems can be interpreted as subtraction or addition problems (with a missing addend), students may write either type of sentence. When students complete the page, ask them to model their representations on the class number line and explain their reasoning.