Subtraction Strategies
Est. Class Sessions: 2Summarizing the Lesson
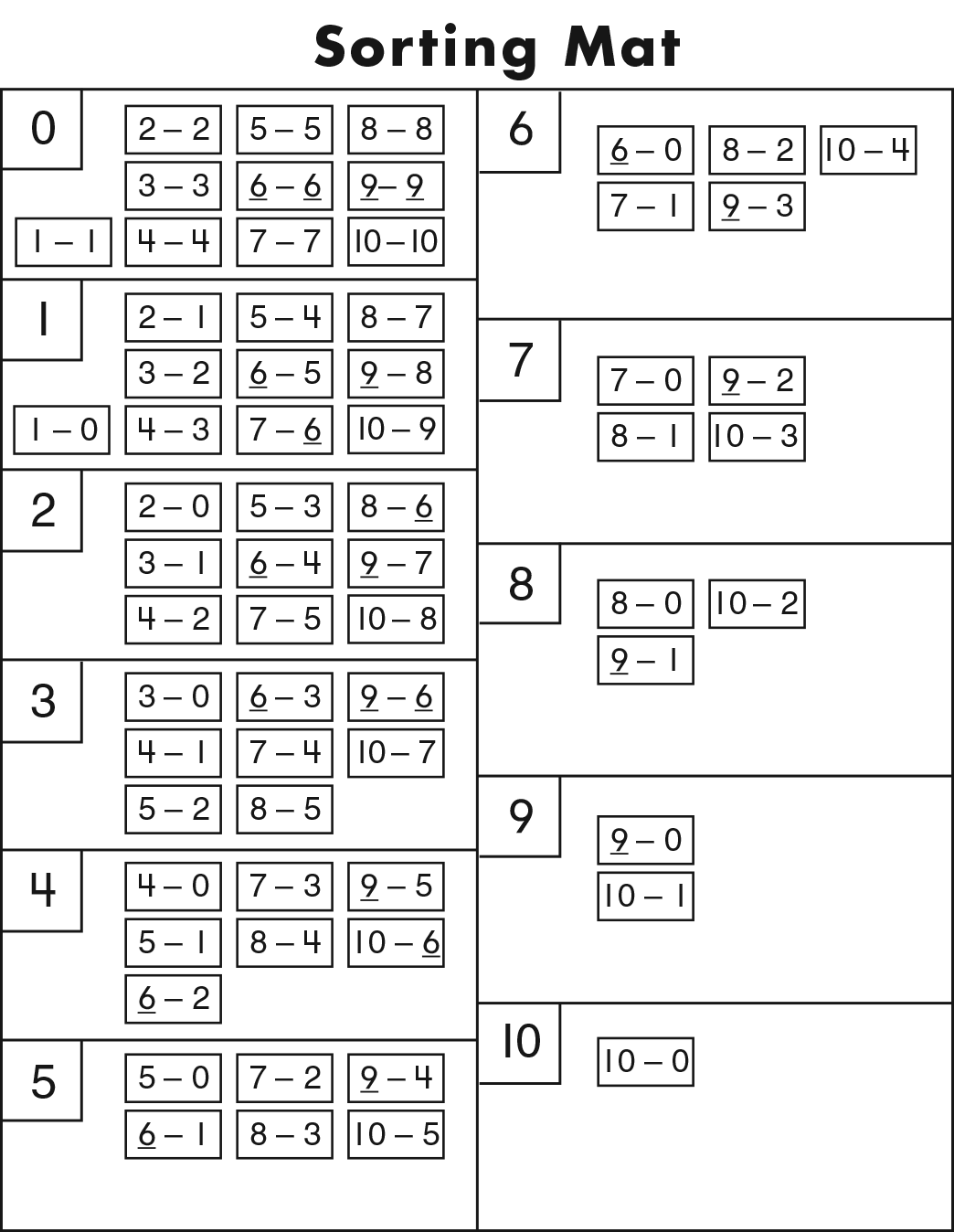
To close the lesson, use the completed subtraction Sorting Mat chart to discuss students' use of subtraction strategies. See Figure 11. The Sorting Mat showing sums from Lesson 1 should also be available for use in the discussion.
Ask:
- Look at all the problems with zero in them. How did you solve them?
- Point out the problems you thought were the easiest to solve. How did you solve them?
- Which problems did you think were the hardest? Let's list them. How did you solve them?
- Did someone solve that problem a different way?
- Can you explain [student name]'s strategy?
- Show how to solve this problem using [student name]'s strategy.
- Show how to use a [number line and the counting-up strategy] to solve this problem.
- Show a partner how to solve this problem two ways.
The Sample Dialog touches on the following topics: subtracting zero; using tools such as cubes, number lines, and ten frames to solve problems; using counting strategies; and using addition to solve subtraction problems.
Teacher: Look at all the problems with zero in them. Can someone read them to me? I will write them on the board. Look for a pattern that will help solve these problems.
Michael: 1 − 0 = 1, 2 − 0 = 2, ... 10 − 0 = 10
Teacher: Michael, where did you find these problems on the chart?
Michael: I found one in each group.
Lee Yah: I see a pattern. All the answers are the same as the first number in the problem.
Teacher: Well said, Lee Yah. Why do you think that works? What does it mean to subtract zero? What are you taking away each time?
Lee Yah: You take away zero which is nothing. So if you take away nothing, then the other number stays the same.
Teacher: Which problems did you think were easiest? How did you solve them?
Jacob: The ones with zero were easiest because you could just say the other number.
Keenya: I could do the ones that took away 1 or 2 the fastest.
Teacher: How did you solve them? Pick a problem like that and show me how you solved it.
Jackie: For 8 – 2, I started with 8 and counted back two on my fingers, 7, 6. It's 6.
Teacher: Which ones do you think were hardest?
Tanya: I got stuck on 9 – 6, so I just used my cubes. I built a train of 9 and took away 6 and got 3.
Teacher: Is Tanya right? Did someone solve that one a different way? What answer did you get?
Nila: We had that problem with the bags, so I started at 6 counted up 7, 8, 9. That's means the answer is 3.
Teacher: So, you agree with Tanya. You two did it different ways but got the same answer. What is another hard problem?
Jessie: 10 – 6 was hard, but I thought to use my number line and it helped.
Teacher: Come show us what you did on the class number line. [Jessie points to 10 on the class number line and shows 6 hops back to 4.]
Jessie: See it is 4.
Teacher: Did anyone use a ten frame to solve this problem? [After a pause] Nicholas, what did you and Roberto decide to do?
Nicholas: We knew to start with ten, so we just imagined that the ten frame was full of dots. Then we pretended to take 6 off. [Nicholas puts a finger in the last box on the ten frame and counts backwards across the bottom row and then covers the last box in the top row with his finger.] So we know that there are 4 boxes left.
Teacher: Roberto, what is a number sentence for that problem?
Roberto: 10 – 6 = 4.
Irma: When we looked at the ten frame, we remembered how we made addition and subtraction sentences.
Teacher: How did that help you solve the problem?
Irma: We knew that 6 + 4 = 10, so 10 – 6 = 4.
Teacher: So Irma used addition to help her solve a subtraction problem. Talk with your partner and think how you can use addition to solve 9 − 5. You might want to look at the addition chart. [After a pause] Michael, how did you and Ming use addition to solve the problem?
Michael: We were stuck, but we looked on the chart at the group of nines. We saw the card that said, "5 + 4 = 9," so 9 – 5 must be 4.
Teacher: That is very good thinking. You have all used some good strategies. You used patterns, tools, counting, and addition to help solve subtraction problems.