Fact Families

Find Facts in Fact Families. Display the Sorting Mat chart for sums from Lesson 1 and the Sorting Mat chart for differences from Lesson 8. Display the Addition Strategies chart from Lesson 1. Each student will need about 10 connecting cubes of one color and 10 cubes of another color.

Review the terms sum and difference. A sum is the answer to an addition problem and a difference is the answer to a subtraction problem. Remind students that they sorted the addition cards by their sums and the subtraction cards by their differences on the sorting mats.

Write "5 + 2 =" on chart paper. Have students model the problem with a train of 5 connecting cubes of one color and a train of 2 connecting cubes of another color as you demonstrate the same. Put the 5 cubes together with the 2 cubes to make a single train.

Complete the addition number sentence on the chart paper as you say, "5 + 2 = 7."

Review the term turn-around fact.

Separate your cube train back into 5 cubes and 2 cubes so that you can give students a visual image of the commutative property by changing the order of the addends in the problem. Ask students to separate their trains back into one of 5 and one of 2.

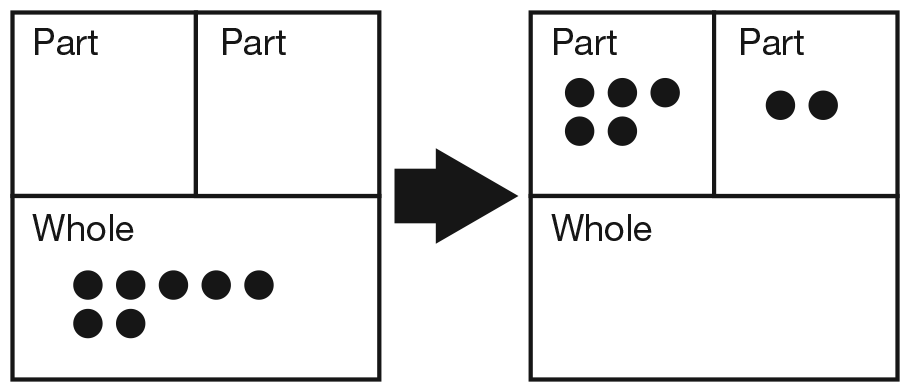
Record 2 + 5 = 7 on the chart paper. Tell students that addition and subtraction have a special relation-ship. They can use addition to help solve subtraction facts. Tell them that sometimes you put cubes together but sometimes you start with the whole and take them apart. Show that you are using the same number of cubes. The whole is the same. In this case, it is 7. Display and use the Part-Whole Diagram and Number Sentences Master and tell students to think about 5 + 2 = 7. Place 7 cubes in the Whole section.

Take 2 away.

Write 7 − 2 = 5 on the chart paper and ask a student to find
7 − 2 = 5 on the Sorting Mat chart for differences from
Lesson 8.

Refer to the part Part-Whole Diagram again and place 7 cubes in the Whole section. Then move 5 into a Part section. See
Figure 1.

Write 7 − 5 = 2 on the chart paper and find 7 − 5 = 2 on the Sorting Mat chart for differences.

Tell students that these number sentences are all related and are called fact families. Draw a box around the fact family. See Figure 2. Since they probably have facts that they "just know," they can increase the number of facts they know if they think of them in fact families.

Have a volunteer choose another fact from the Sorting Mat chart for sums from Lesson 1. Repeat the activity with this fact using trains of connecting cubes and the Part-Whole Diagram to find the addition turn-around fact and related subtraction facts. Record the related number sentences on chart paper.

Solve Facts and Sort by Fact Families. Distribute one of the pages from the Fact Families 1–6 Masters to each student pair. Students will solve the facts on one of the pages using connecting cubes, ten frames, or number lines as needed. After they have solved the problems, tell students to cut along the lines to separate the facts on the page. Ask students to sort the facts into fact families. They should group and glue the facts in fact family clusters onto a piece of paper.