Review Number Lines. In this lesson, students use number lines to represent fractions. This helps reinforce the fact that fractions are numbers, just as whole numbers are. Students often think of a fraction as two separate numbers, a numerator and a denominator, and working with number lines helps overcome this misconception. The number line also provides a visual representation of numbers in increasing order that helps students understand the relative size of fractions.
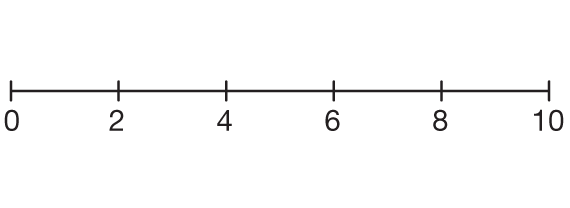
An important concept for students to understand when using number lines is that all numbers have a place on the number line even if they are not labeled. To help develop this concept, draw a number line on which not all whole numbers are labeled. For example, draw a number line from 0 to 10 that shows only even numbers, as in Figure 1.
Ask questions that help students understand that the number line has positions for all numbers:
- Are there any numbers you can label on the number line between the numbers I have written? (Yes, you can label the odd numbers.)
- Where do the odd numbers belong? (Put 1 between 0 and 2, 3 between 2 and 4, etc.)
- Where can you put the fraction 1/2 on this number line? (halfway between 0 and 1)
- How about 11/2 ? (halfway between 1 and 2)
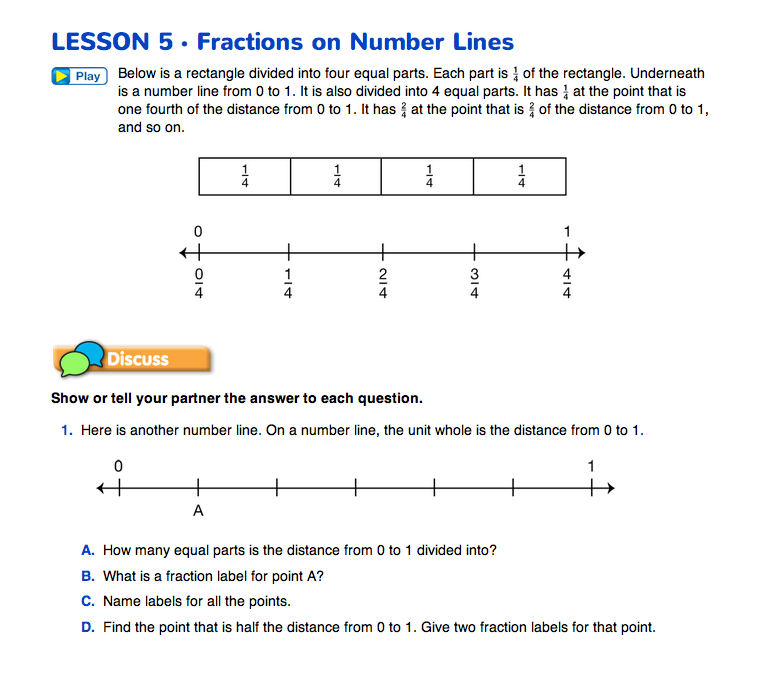
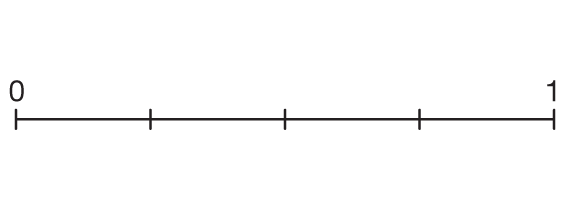
Next draw a number line from 0 to 1. Divide the segment into four equal parts by making equally-spaced marks between 0 and 1 as in Figure 2.
Help students label the number line by asking:
- Here is a number line from 0 to 1. What fraction of the line is each part? (one-fourth)
- How do you know? (The lines divide the space into four equal parts so each part is one-fourth.)
- How should you label the first mark after zero?
(Write 1/4 beneath the first mark.)
- What does the denominator in the fraction 1/4 tell you? (The denominator tells the number of equal spaces the line is divided into.)
- What does the numerator in the fraction 1/4 tell you? (The numerator tells us that it is the first mark we are interested in. One-fourth tells us the fraction of distance from 0 to 1 that the first mark represents.)
- Where should you write 2/4? (at the mark that is 2/4 or one-half of the distance from 0 to 1)
- How do the numbers in the fraction help you know where to write 2/4? (The numerator tells us that we are looking at the mark on the line where 2 of the four equal spaces ends.)
- How should you label the third mark after zero? (Write 3/4 beneath the third mark.)
- What is another name for the mark that is labeled “1”? (4/4)
- What is another name for the mark that is labeled “0”? (0/4)
Label Fractions on Number Lines. Ask students to look at the rectangle on the first page of the Fractions on Number Lines pages in the Student Guide.
To help them compare the rectangle and the number line below, ask:
- How are the rectangle and the number line alike? (Both are divided into the same number of equal parts. The ends of each part of the rectangle match the ends of each part of the number line.)
- How are they different? (The parts of the rectangle are all labeled the same. Each is labeled 1/4. But on the number line, the labels count up 0/4, 1/4, 2/4, 3/4, 4/4.)
- Do the labels mean the same thing? (No, the labels on the rectangle show how big each part is. They show that each part is 1/4 of the whole rectangle. The labels on the number line show what fraction of the distance from 0 to 1 the mark is.)
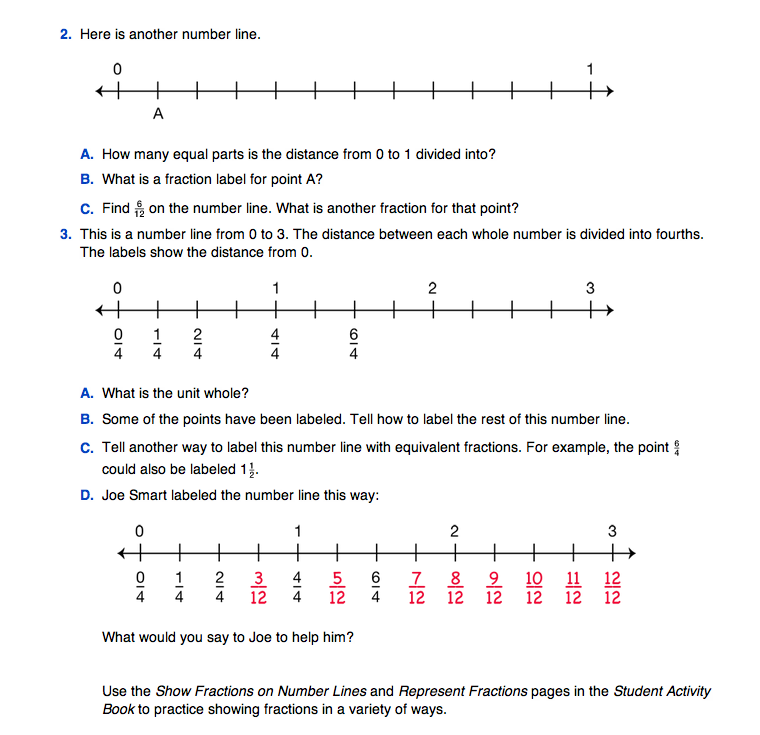
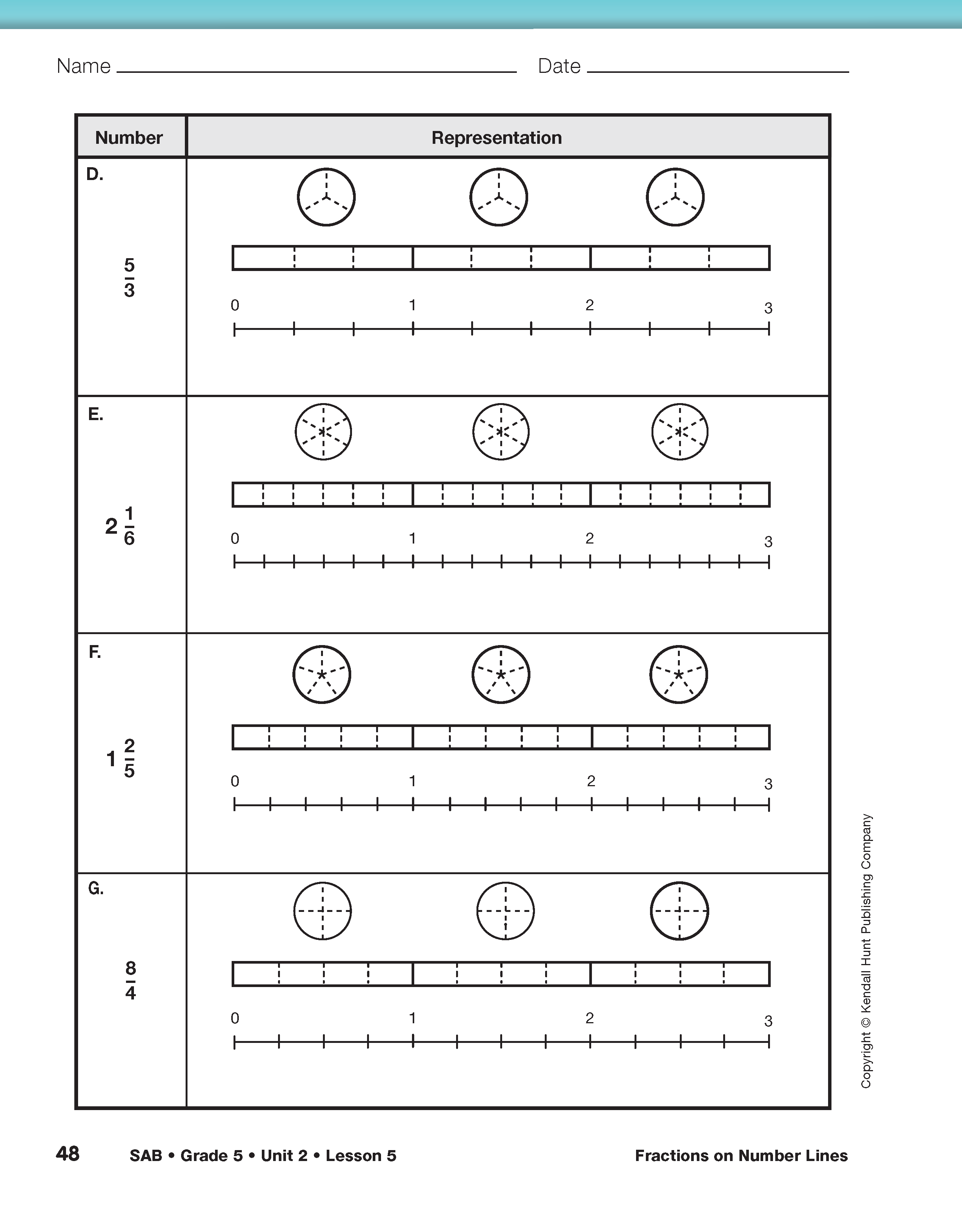
Assign Questions 1–3 in the Student Guide to partners, or have the whole class answer them together. In these questions, students decide how to label the number lines based on the number of equal parts into which the interval from 0 to 1 is divided. Question 3 involves a number line from 0 to 3, but the unit whole is still the interval from 0 to 1.
A common mistake for students to make is to think that the number of marks on the number line represents the denominator, despite the number of whole numbers on the number line. In Question 3D, Joe counts twelve marks dividing the 0 to 3 number line, and labels the points as twelfths instead of fourths.
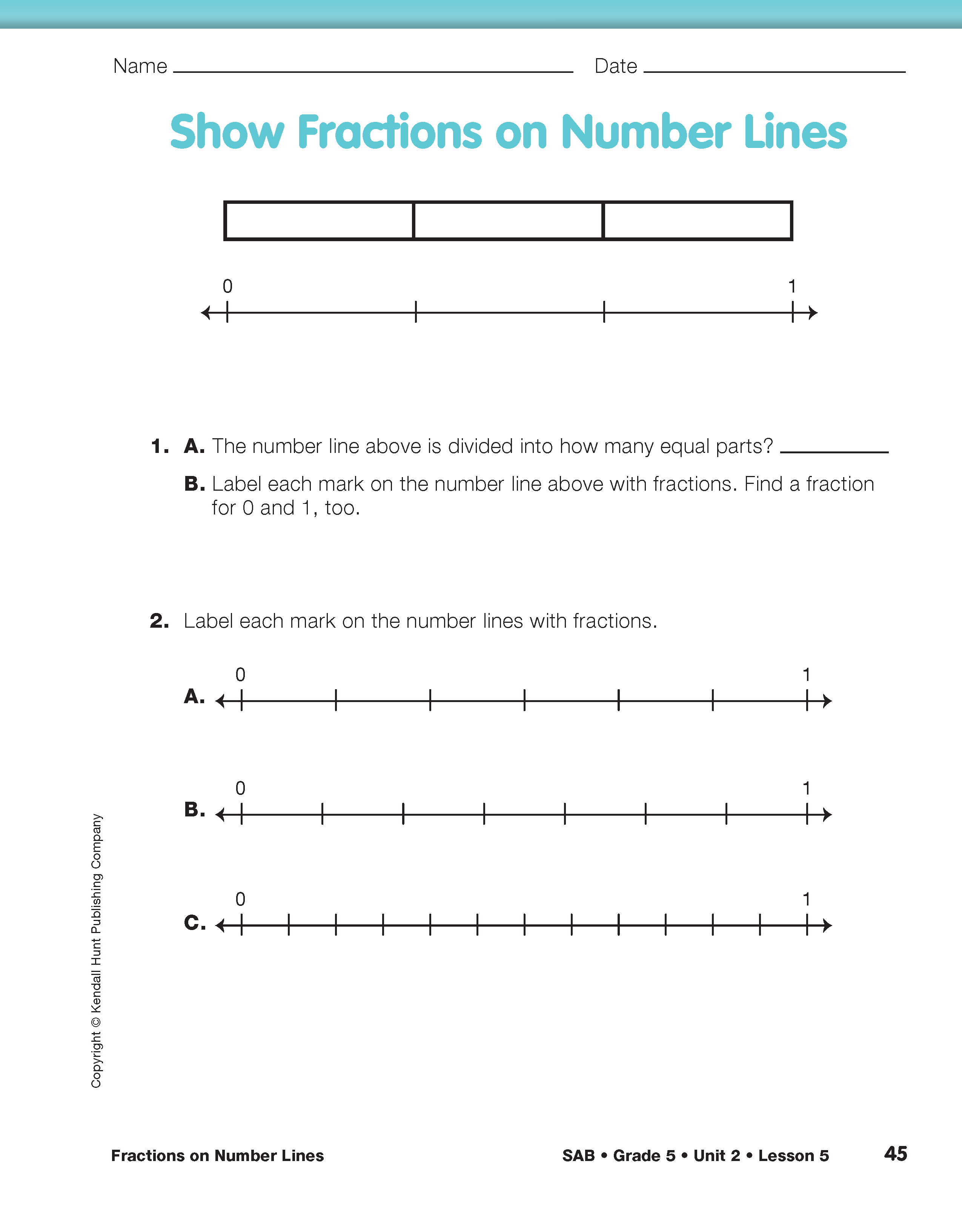
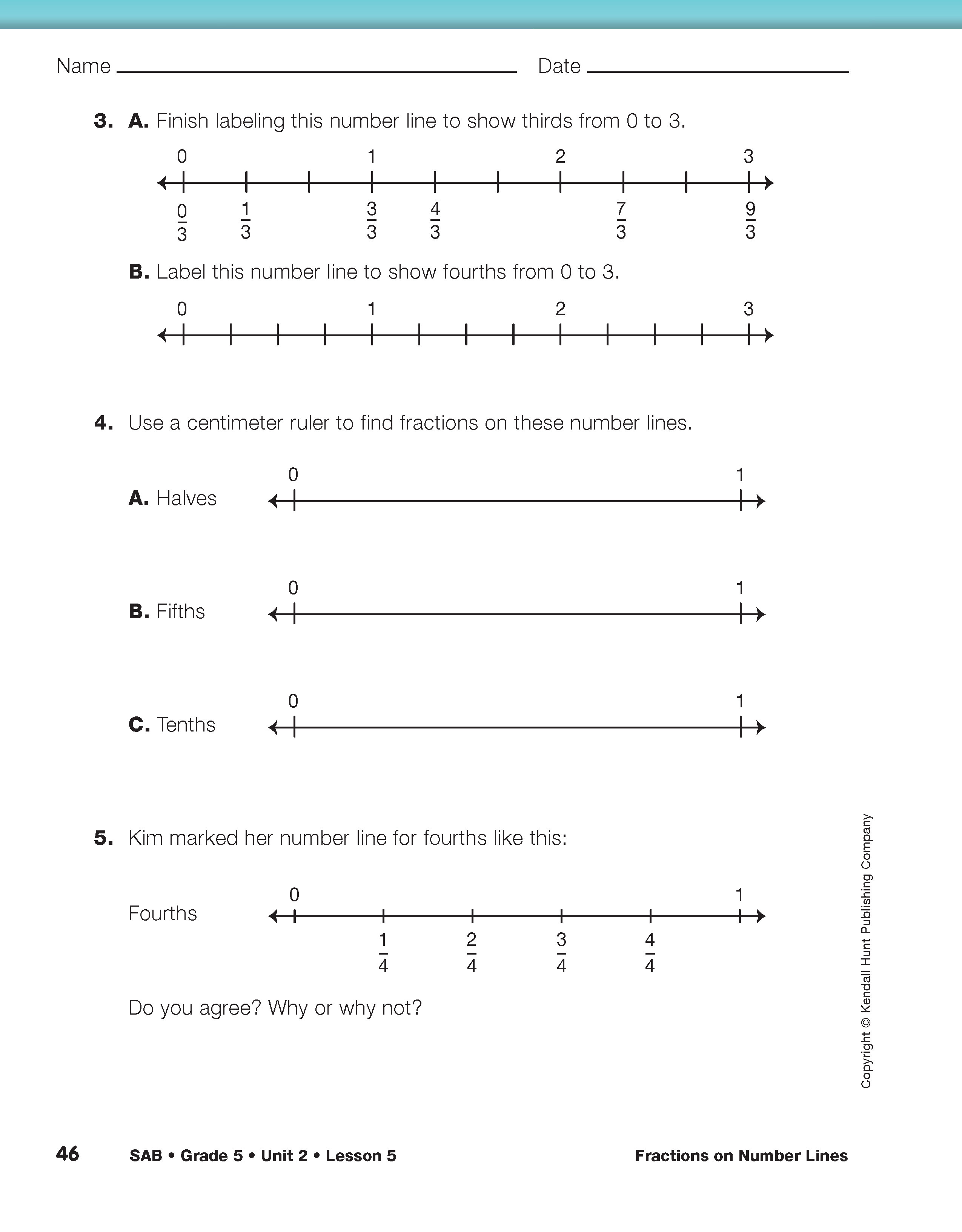
Next, ask students to complete the problems on the Show Fractions on Number Lines pages in the Student Activity Book. In Questions 1–3, students label marks on number lines with fractions. In Question 4, they first divide the interval into equal parts and then label the line with fractions. A centimeter ruler will help make the divisions equal. Students will find that the interval from 0 to 1 is 10 cm long, which will help them to divide the line into halves, fifths, and tenths.
Question 5 addresses a common misconception. Instead of dividing a number line into four equal parts to show fourths, Kim draws four marks on the line between the whole numbers 0 and 1 and erroneously creates fifths.
- In Question 5, did Kim show fourths on the number line? (No, she showed fifths.)
- What would you say to help Kim? (Possible response: She made four marks on the line instead of dividing the number line into four equal parts. When she is done, she should check to make sure her fourths number line has only four equal parts from 0 to 1.)
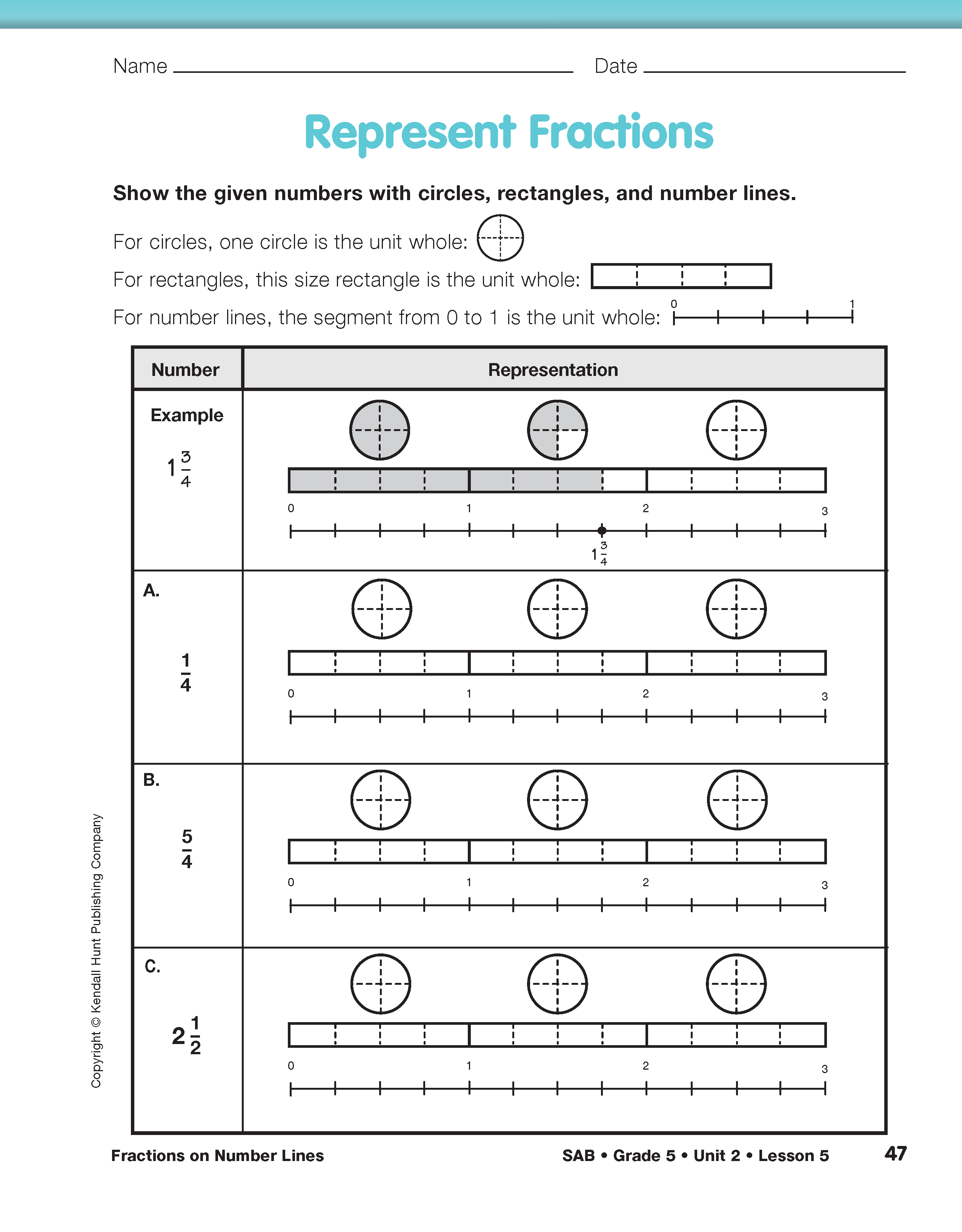
Direct students' attention to the example problem on the Represent Fractions pages in the Student Activity Book and ask:
- In the example, what is the unit whole for the number line? (the segment from 0 to 1)
- How many unit wholes are on the number line?
(3 whole units)
- Each unit whole on the number line is divided into how many equal parts? (four)
- What is the name for each part? (one-fourth)
- How is 13/4 represented in a variety of ways in this example? (On the number line, the mark that is one whole unit and then 3 more marks past the 1 is 13/4; On the circles, 7 of the circle fourths are shaded to show 13/4; On the rectangle, one whole unit on the rectangle and then 3 more are shaded to show 13/4.)
- Is there another name for 13/4? (7/4)
- How do you know? (Possible response: I can count the number of fourths shaded in the representation of 13/4. Seven fourths are shaded. Or, I know 4 fourths make 1 plus 3 more fourths is 7/4.)
Assign the Represent Fractions pages for students to complete individually.
Use the Represent Fractions pages in the Student Activity Book to assess students' abilities to represent and identify fractions (e.g., proper, improper, mixed number) using area models and number lines [E1].
To provide targeted practice, make copies of the Representing Fractions in Many Ways Master. Allow students to choose from a prepared list of a variety of fractions (proper, improper, and mixed number such as 2/3, 11/5, 21/4) to represent with the circle, rectangle, and number line representations on the page. Alternatively, they can choose fractions on their own to represent several different ways.