Lesson 6

Using Multiplication Strategies

Est. Class Sessions: 2

Developing the Lesson

Part 2: Multiplication with Larger Numbers

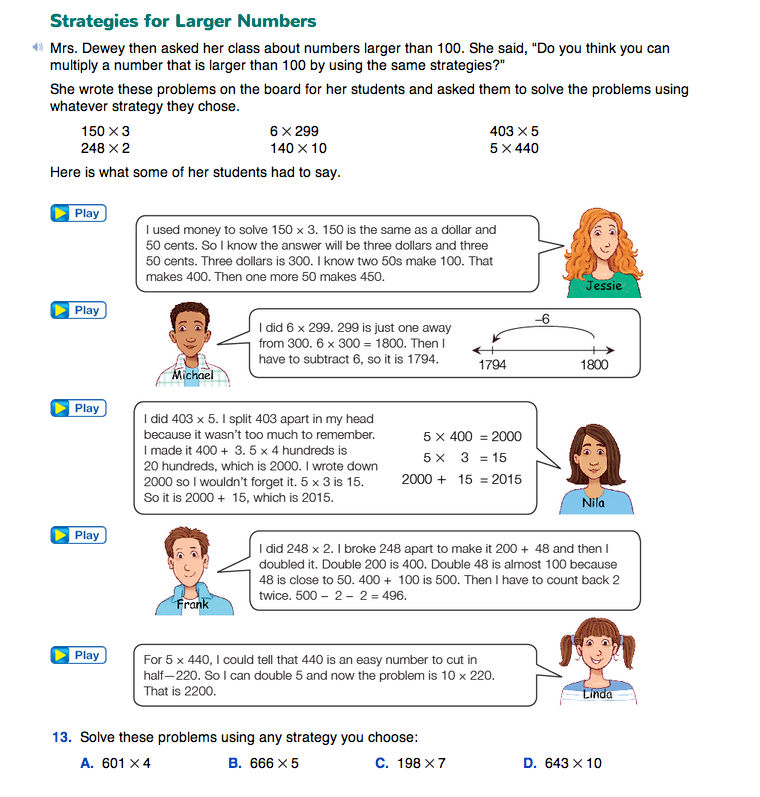
This part of the lesson extends students' thinking to problems involving multiplication of 3-digit by 1-digit numbers. Before reading the vignette in the Strategies for Larger Numbers section in the Student Guide, write the following six problems from the vignette on the board:
        150 × 3           6 × 299         403 × 5
        248 × 2         140 × 10         5 × 440

Ask:

X
  • Can you use the same strategies and methods—both mental math and paper-and-pencil—that you used for 2-digit multiplication to solve these problems?

Have them work in pairs to discuss and solve the problems. Remind them that sometimes a few quick notes are helpful for keeping track of steps or partial products when solving a problem in their heads.

Have a brief discussion of student strategies and methods. Then have students read the vignette on the Student Guide pages and the strategies used by the students in Mrs. Dewey's class. Guide students to see that the same strategies they used for 2-digit by 1-digit problems can work with larger numbers.

Ask:

X
  • Describe the strategies in your own words.
  • What do you think of the strategies these students used?
  • Do they make sense to you? Which ones do and which ones do not? Why?
  • Can you explain Jessie's strategy using your own words or show it on the board? Michael's strategy?
  • Can you show me how to use Nila's strategy to solve 105 × 4?
  • Do you think Linda's strategy would have worked well for Jessie's or Michael's problem? Why or why not?
X

A variety of strategies are introduced with a variety of problems because some strategies better suit a problem. For example, an efficient strategy to solve 444 × 5 is to multiply by ten and then divide the answer in half. “Thinking money” is not an efficient or helpful strategy for this problem.

For Question 13, ask students to demonstrate their strategies. Question 13A can be solved by breaking into hundreds and ones; 13B can be solved by doubling 5, multiplying by 10, and then halving the answer; and 13C can be solved by rounding up 198 to the easier number 200, multiplying by 7, then counting back by two 7 times. Question 13D can be solved by using patterns with zero.

If you have not assigned all the problems in the Homework section, you may assign the rest now.

Student Guide: 282
Answers
X
SG_Mini
+
Student Activity Book:
Master: