Divisibility Rules

Divisible by 6. To explore divisibility by 6, students need their copies of the 100 Chart from Part 1 of this lesson. The multiples of 2 should be circled in blue. The multiples of 3 should be marked in red.

Questions 16–17 explore divisibility by 6. Students may notice that the numbers divisible by both 2 and 3 are also divisible by 6. When answering Question 16B, students should recognize that there is no need to mark the multiples of 6 on the chart because they are already marked with blue circles and red Xs.

Since a number that is divisible by 6 is one that is also divisible by 2 and 3, students may predict that the number 12,345,678 is divisible by 6 (Question 16C). Checking this on the calculator will prove them to be correct.

For further practice, have students identify which of the following numbers are divisible by 6.

116      108      123      1008

108 and 1008 are divisible by 6.

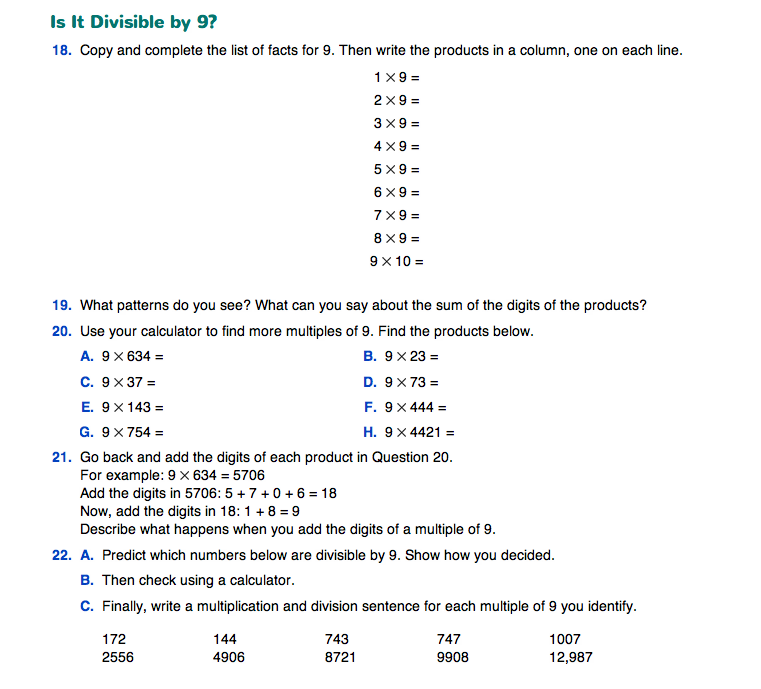
Divisible by 9. After students have explored the divisibility rules for 2, 3, and 6, have student pairs complete Questions 18–22 in the Student Guide. These questions explore divisibility by 9. Some patterns students may discover in Question 18 are as follows:

• When the products are listed in a column, as below, the digits in the tens place count up by ones (0, 1, 2, 3 …) and the digits in the ones place count down by ones (9, 8, 7 …).
  9
  18
  27
  36
  45
  54
  63
  72
  81
  90
• The sum of the two digits in each of the products listed above is 9. In fact, the sum of the digits of any multiple of 9 is also a multiple of 9.

Have students work with other multiples of 9 in Questions 20–21 to discover that this pattern is consistent. The process of adding digits can be repeated until nine itself results. This is illustrated in Question 21. In Question 22, students may use this pattern to predict whether a number is a multiple of 9.