Lesson 10

Break-Apart Products with Larger Numbers

Est. Class Sessions: 2–3

Developing the Lesson

Part 2. Represent Break-Apart Products

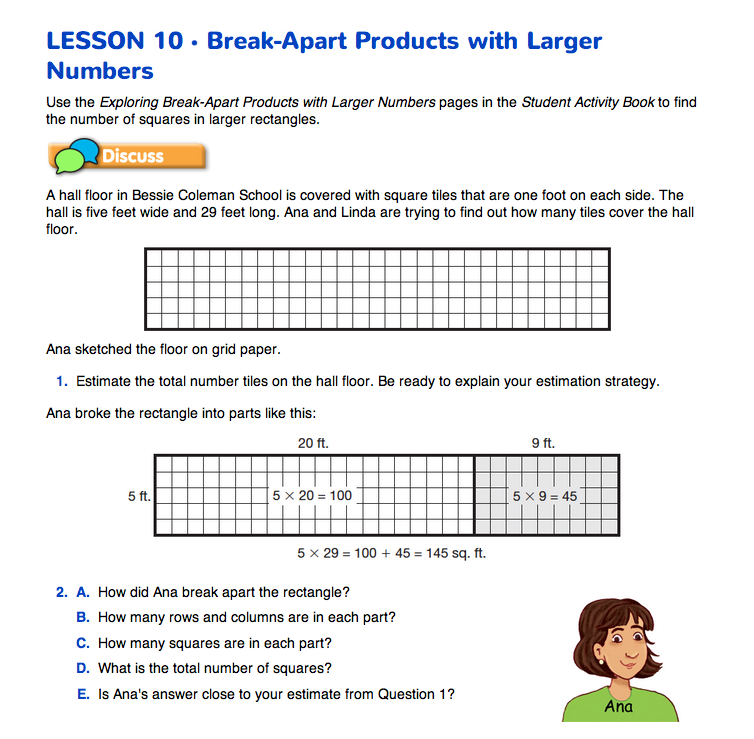
Direct students' attention to the Break-Apart Products with Larger Numbers pages in the Student Guide, Question 1.

Ask:

X
  • What is a quick way to estimate the number of square feet in the 5 × 29 foot hall in Question 1 without using paper and pencil? (A likely strategy is to multiply 5 times 30 for an estimate of 150 square feet. Students may choose to get a closer product by subtracting one 5 to mentally arrive at an exact answer of 145 square feet.)

Have student pairs discuss Question 2. Talk with students to be sure that they can match Ana's number sentences with the picture.

Ask:

X
  • Why do you think Ana chose to divide the rectangle into 20 and 9? (Possible response: Multiplying by tens and adding tens are easy.)

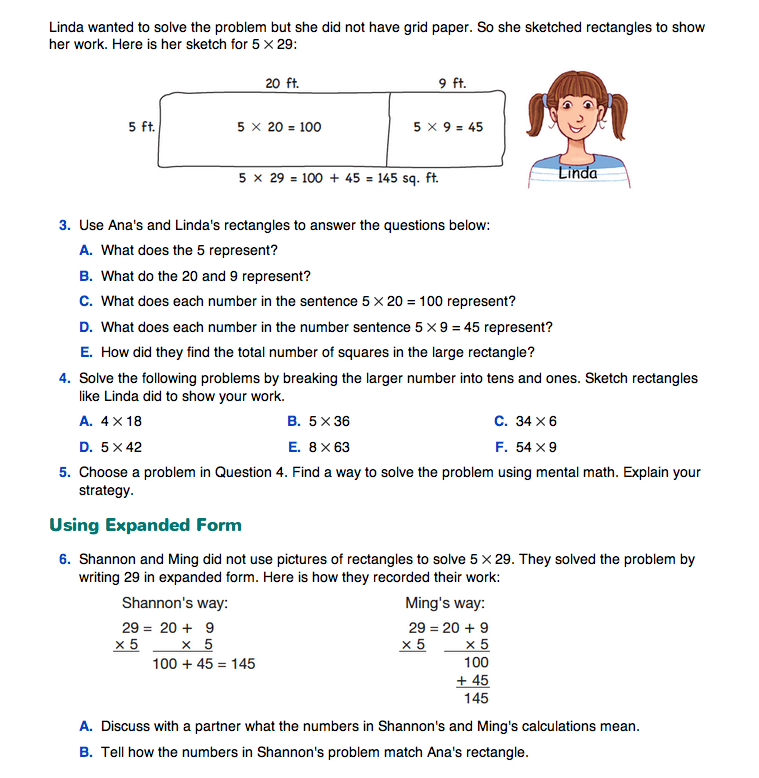
For Question 3, students compare Ana's rectangle with a grid to Linda's sketch without a grid. As pairs discuss the questions, check to see that they understand that the 5 still represents the number of squares along the width and that 20 and 9 represent the number of squares along the length of the parts. The numbers 100 and 45 represent the number of squares in each part, and they are added together to find the total.

Students practice using rectangles to represent the break-apart method in Question 4. They consider a mental math strategy for one problem in Question 5. For example, students may visualize dividing a 5 × 42 rectangle into two equal parts for Question 4D. Then mentally calculate:

5 × 21 = 105
105 + 105 = 210

Student Guide: 103 104
Answers
X
SG_Mini
+
X
SG_Mini
+
Student Activity Book:
Master: