Lesson 8

Prime Factors

Est. Class Sessions: 2

Developing the Lesson

Part 1: Finding Prime Factors

X

If necessary, review the terms that are involved in the tasks in this lesson. For example, ask:

  • If I write this number sentence, “36 = 4 × 9,” which of the numbers are factors and which is the product? (36 is the product and 4 and 9 are factors.)
  • What does it mean to “write 36 as a product of three factors”? (Write the same kind of number sentence, but multiply three numbers instead of two. For example, 2 × 2 × 9 = 36.)

Find Factors of Composite Numbers. Have students work with a partner on this task:

  • Find as many different ways as you can to write 36 as a product of three or more factors.

Possible responses include:
          36 = 3 × 4 × 3
          36 = 3 × 2 × 6
          36 = 9 × 2 × 2
          36 = 2 × 2 × 3 × 3

Note that the last example is a number sentence that renames 36 as a product of primes. If some students find factors that are all prime numbers, ask them to tell how they found their answers.

If none of your students find all prime factors, choose one of their answers and ask:

X
  • Can the factors in your number sentence be factored into smaller numbers?

For example, in the sentence 36 = 9 × 2 × 2, the 9 can be factored into 3 × 3. The sentence then becomes
36 = 3 × 3 × 2 × 2.

Ask students to work with the same partner to complete the following:

  • Write 24 as a product of at least three factors. (Possible responses include
    2 × 3 × 4, 2 × 2 × 6, 2 × 2 × 2 × 3.)

As pairs of students finish writing their products, ask:

X
  • Try to write 24 as a product of factors that are all prime numbers (that cannot be factored any further).
    (2 × 2 × 2 × 3 = 24)

Have students share their number sentences with the class.

After at least two students share their number sentences, ask:

X
  • Are the number sentences the same? (The correct number sentence is 24 = 2 × 2 × 2 × 3, though the factors need not be in that order.)
  • Does it matter if the order of the factors is not the same? (No, because of the turn-around rule or the commutative property.)
  • How can you tell that all the factors in these number sentences are prime numbers? (Students may say that the factors cannot be factored any further, or that each factor cannot be divided by any number other than one and the number itself.)

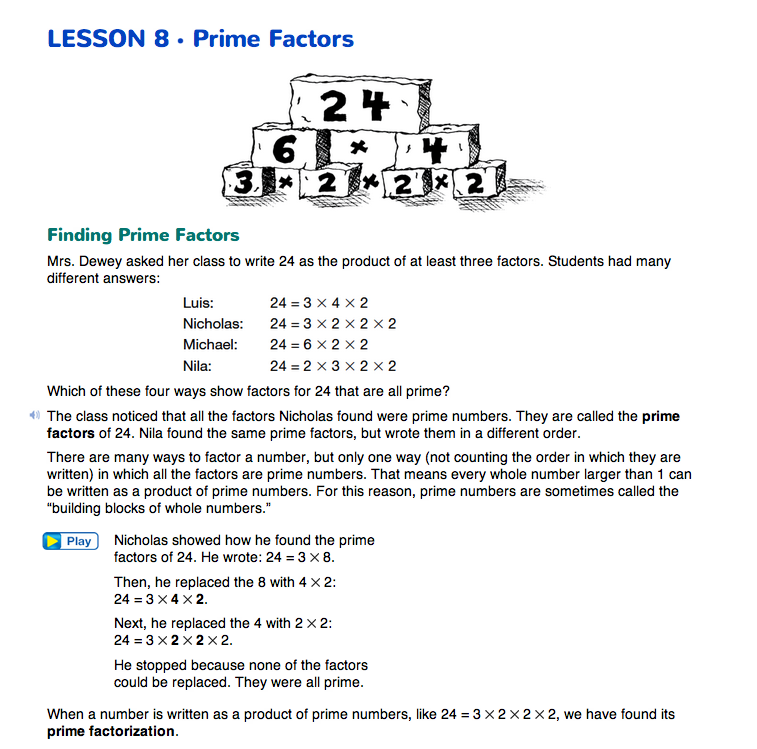
Ask students to read the vignette in the Finding Prime Factors section of the Student Guide. After reading Nicholas's explanation of his strategy, ask students to compare Nicholas's strategy to their own.

Make Factor Trees. Show the class how to use a factor tree to organize the work in a search for prime factors. Draw the factor tree that Mrs. Dewey drew on the board as shown in the Student Guide. Have a student show how each step of the factor tree matches with each of Nicholas's steps. Then ask a student to explain each step of Nila's factor tree. Ask another student to finish the factor tree that John started in Question 1. See Figure 1.

The Prime Factors pages in the Student Guide give examples of factor trees for 24, starting with 3 × 8, 6 × 4, and 2 × 12. For additional class practice, work together to make factor trees for 30 and 40.

When students are ready, ask them to answer Questions 2–3, which provide additional practice with factor trees. Students can discuss their solutions with partners.

X

Use Check-In: Question 3 on the Prime Factors page in the Student Guide to assess students' abilities to find the prime factorization of a number [E6].

In Question 4, students distinguish between finding all the factors of a number and finding the prime factorization of a number. Clarify for students the meaning of each. See Content Note.

X

“Finding all the factors of a number” refers to naming the numbers found in all of the unique factor pairs of a number. For example, the factors of 18 are 1, 2, 3, 6, 9, and 18, since 18 can be written as 1 × 18, 2 × 9, or 3 × 6 (and the reverse of each).

The prime factorization of a number, on the other hand, is the number written as a product of prime numbers. If the order of the factors is not considered, there is only one way to write a number as a product of primes. For example, the prime factorization of 18 is 2 × 3 × 3.

The prime factorization of a prime number is just the number itself. For example, the prime factorization of 7 is 7.
(It is not 1 × 7, since by definition, 1 is not a prime number.)

Use Exponents. Students practice using exponents to write prime factorizations of numbers in Questions 5 and 6.

Assign the Factors and Primes section of the Homework page in the Student Guide after Part 1.

Student Guide: 97 98 99
Answers
X
SG_Mini
+
X
SG_Mini
+
X
SG_Mini
+
Student Activity Book:
Master:
A factor tree for 24 for Question 1
X
+