Lesson 9

Product Bingo

Est. Class Sessions: 2

Developing the Lesson

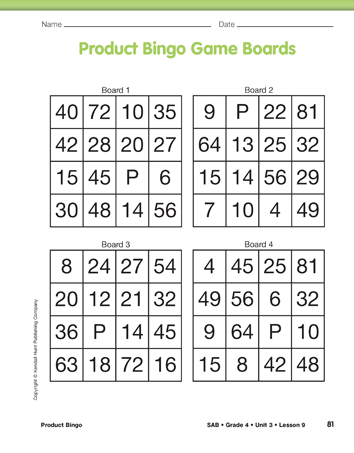
Play Product Bingo. Begin by discussing the directions on the Product Bingo pages in the Student Guide. Demonstrate a few turns with the class to clarify how the game proceeds.

Ask students to play Product Bingo for 30 minutes. They may play several games in that time. Remind students to switch callers and game boards for each new game.

Ask the caller to record which Product Bingo game board won in each game.

Have each group discuss Questions 1–6 on the Product Bingo pages in the Student Guide.

X

Board 3 typically wins most often because it has five products (18, 12, 24, 36, and 16) that can be spun more than one way. Board 2 can never win because 22, 13, 29, and 7 have no factor pair on the spinner, so those numbers can never be marked. It is possible to win the game using Boards 1 and 4, but less likely than with Board 3, since each product on Boards 1 and 4 can be spun only one way. Make sure students switch game boards for each new game.

X

Pay attention to the discussions of students in their small groups. If you note some groups of students having trouble identifying why one board may be better than another, ask scaffolding questions such as the following:

  • Which products did you get on the winning boards?
  • Did you get some products more than once? Which ones?
  • What 2 numbers did you spin to get those products?
  • Did you get those products spinning any other numbers?
  • What do you call the numbers that you multiply to get a product?
  • Were there some products on your boards that you never got? Which ones?
  • What are the factors of those products that you never got?

Discuss Game Boards. Engage the whole class in a discussion of winning and losing game boards. See the Sample Dialog. Ask students to share the results of which boards won and display the class totals on the board. See Figure 1.

X

Teacher: Jerome, what do you think about our class totals?

Jerome: Board 3 is the lucky board. Everyone wanted that board after awhile.

Teacher: Why do you think it was lucky? Do you think a different board might be lucky tomorrow?

Jerome: It was lucky because it won the most.

Teacher: But why do you think that happened?

Jerome: We kept getting 12 and 24 and none of the other boards have those numbers.

Teacher: Why were those good products?

Maya: Because you could get them lots of ways. Twelve, you could get with 2 × 6 and with 3 × 4.

Teacher: Twelve and 24 are products. Do you have a name for those other numbers you said, 2, 6, 3, 4?

Maya: They're the factors. Factors of 12.

Teacher: Good. Yes, 2, 3, 4, and 6 are all factors of 12. What is the difference between Board 3 and another board, say, Board 2? Look at the products on those boards.

Ana: On Board 2, you have products that are never going to win, like 22 and 7.

Teacher: Why are those products never going to win? Don't they have factors?

Ana: Seven doesn't.

Teacher: Seven doesn't have any factors?

Ana: I mean it's only got two factors, 1 and 7. It's a prime number.

Teacher: That's right, Ana, it's a prime number, but why do you say it can never win?

Ana: Because 1 isn't on the spinner so you can never spin it.

Teacher: What about 22? Why will that never win? Is it a prime number too?

Luis: I don't think it is a prime number.

Teacher: Why don't you think it is prime?

Luis: Because you can divide it. It ends in 2.

Nila: It's 11 times 2.

Teacher: What did you say, Nila? What are the factors of 22?

Nila: 11 and 2. Also 1 and 22.

Teacher: Okay, so it's not a prime number. So why will it never win?

Nila: Because 2 is the only one that is on the spinner. The spinner doesn't have 1 or 11 or 22, so you can never spin a 22.

Encourage students to examine the factors of the numbers on each board. The board that is most likely to win is the board whose numbers have the greatest number of factors that are on the spinner. For example, Board 2 has the number 13 on it. 13 is prime. It has two factors, 1 and itself. The factors, 1 and 13, are not on the spinner, so the number 13 on Board 2 can never be filled in. However, a number like 12 on Board 3 can be filled in by spinning 2 and 6, or 3 and 4.

Students can make a list of the possible factors for each number on the boards (keeping the numbers on the spinner in mind) to help them find the board that is most likely to win. Assign one game board to each group. Then use the result to help students compare game boards.

Student Guide: 101 102
Answers
X
SG_Mini
+
X
SG_Mini
+
Student Activity Book: 81
X
SAB_Mini
+
Master:
Sample table for recording winning and losing boards
X
+