Lesson 1

Multiplication and Rectangles

Est. Class Sessions: 2–3

Summarizing the Lesson

Multiples, Primes, and Square Numbers. After the class chart is complete, ask students to work on Questions 7–16 in the Multiples, Prime Numbers, and Square Numbers sections in the Student Guide.

A number is a multiple of 2, for example, if it equals 2 times another whole number. If you can make a rectangle with 2 rows for a number, then it is a multiple of 2. A number is a multiple of 3 if it can be made into a rectangle with 3 rows. Also, multiples of a number are the numbers you say when you skip count by that number (Questions 7–10).

Prime numbers are defined as numbers that are larger than one and have only one rectangle (Questions 11–13). We will return to prime numbers in Lesson 3 and define them in terms of their factors.

X

0 is a Multiple. Zero is a multiple of every number since every number times 0 equals 0. n × 0 = 0. However, when 0 is the multiple, it is obvious that a rectangle cannot be made with 0 tiles.

One is Not Prime. Since a prime number is conventionally defined as a number with exactly two factors (itself and one), one is not considered to be prime. Note that two is prime since its only factors are itself and one. Two is the only even prime number.

Composite Numbers. During the discussion of prime numbers, the question sometimes arises of what to call numbers that are not prime numbers, the ones that have more than one rectangular array. Tell students that these are called composite numbers. The definition of a composite number is a number that has more than two distinct factors. All whole numbers that are not prime numbers and are not one (1) are composite numbers.

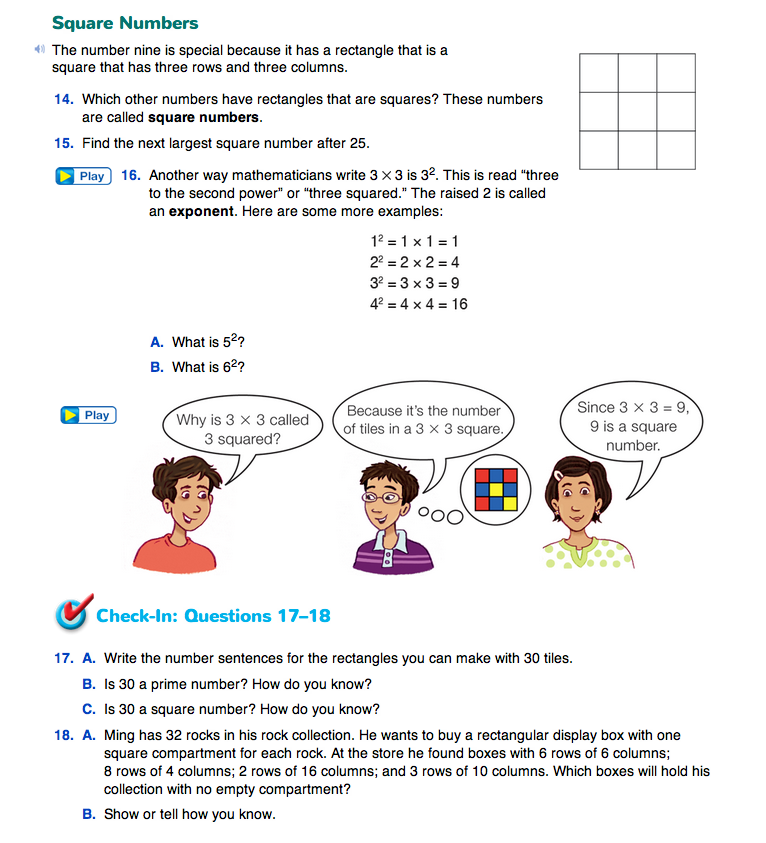
A nice feature of this activity is that students will see clearly why the square numbers have their names (Questions 14–16). Exponents are introduced as a way to write square numbers: 5 × 5 = 52. We will return to exponents in Lesson 7.

Students may want to color code the class chart to identify prime and square numbers.

After students have completed Questions 7–16, assign Check-In: Questions 17–18 to students as a way to assess their understanding of this lesson.

X

Use Check-In: Questions 17–18 to assess students' progress toward representing multiplication and division problems using rectangular arrays [E1]; indentification of prime numbers [E4]; and identification of square numbers [E5].

The Workshop in Lesson 6 provides targeted practice.

Student Guide: 68 69
Answers
X
SG_Mini
+
X
SG_Mini
+
Student Activity Book:
Master: