Workshop: Factors, Multiples, and Primes

Review student work and progress from previous lessons to guide instructional decisions about the following expectations:

E1.

Represent and solve multiplication and division problems using rectangular arrays.

E2.

Determine whether one number is a multiple of another number.

E4.

Identify prime numbers.

E9.

Break products into the sum of simpler products to solve multiplication problems (applying the distributive property of multiplication over addition).

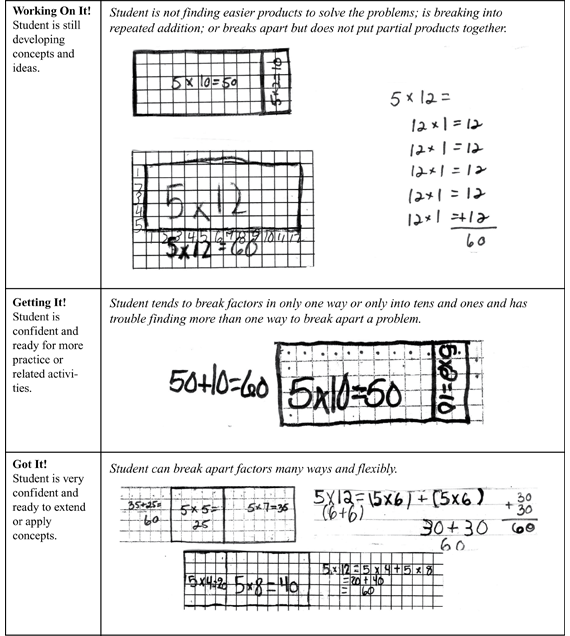
In Part 2 of the lesson, students will identify their level of confidence with a particular concept and choose problems from a Workshop Menu. See the TIMS Tip for more about Workshop Menus. Use your own evaluation of students' progress to help guide their choices. Review the student work samples in Figure 2 to interpret and guide students' confidence levels with the use of the break-apart multiplication strategy.

Plan to organize the class into pairs or small groups based on the problems students select. These groups may change as the workshop progresses.

In Part 3, students revisit the Floor Tiler game that was first played in Lesson 4. See Lesson 4 for instructions if students have not played this game before. Use the Floor Tiler Grid Paper Master to provide additional copies for students that played this game in Lesson 4. If students have not played this game before, you may want to do Part 3 with the class first since there are many references to the game in this lesson.