Review Notation for Parts and Wholes. In
Questions 6–24, students connect the circle pieces to their work using fraction strips and the Fraction Chart in Lessons 1–5. Have students continue to work in pairs to solve the problems throughout the lesson.
Developing Fraction Sense. Questions 6–24 address fraction concepts that provide a basis for developing procedures such as finding equivalent fractions, comparing fractions, and computing with fractions. As they solve the problems, students are also developing visual images of fractions, so that they have a sense of the size of fractions. In previous grades, students learned that equal fractional parts of a unit whole are the same size. Here, they also solve problems based on the idea that the same fractional parts of different-sized unit wholes are not equal. See the Background for more information on these concepts and possible misconceptions.
In some questions, students write fractions based on the red circle as the unit whole, and compare the fraction size to the 1/2 benchmark. In other questions, the unit whole is a piece or shape other than the red circle. Students are asked both to identify the fractional part when given the unit whole and to identify the unit whole when given a fractional part.
The text before Question 6 briefly reviews what is signified by the numerator and the denominator in a fraction. Read this text aloud in class and have the students model it with their circle pieces as you or a student reads.
Ask follow-up questions to check for understanding:
- [Show 3 yellow pieces.] What fraction of the red circle is this? (3/4)
- Why is 4 the denominator? (Because 4 yellow pieces fully cover the red circle. They divide the circle into 4 equal parts.)
- How do you decide what the numerator is? (We are looking at only three of the four pieces that make up the whole.)
- How do you write it as a fraction? (3/4)
- How do you write it in words? (three-fourths)
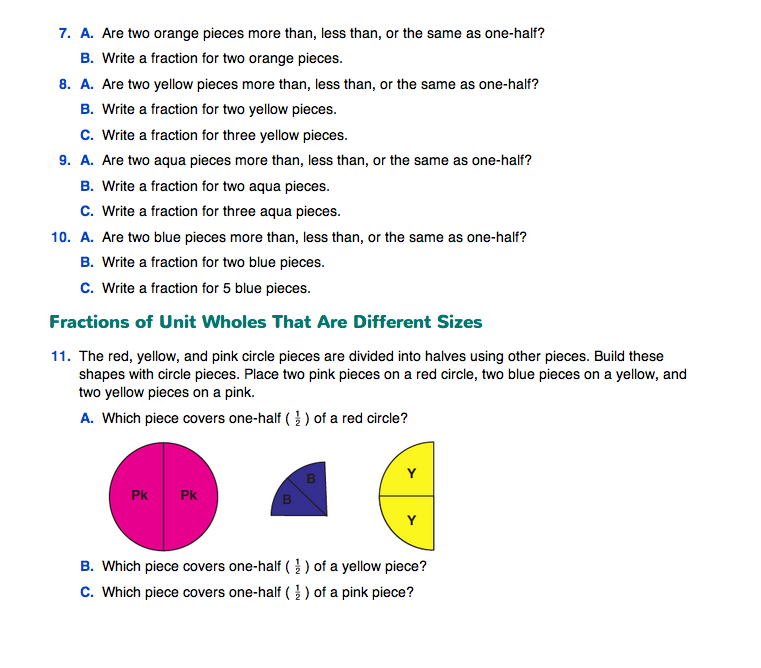
- What piece is the unit whole? (red circle)
Assign Questions 6–10 to student pairs.
Fractions of Unit Wholes That Are Different Sizes. Research in classrooms has shown that some students may have difficulty when the unit whole is not the red circle, such as when the unit whole is identified as the pink piece. Questions 11–13 explore this issue. Remind students that in the past they have found a half of many different things, with many different sizes and shapes.
Hold up two different-sized books and ask:
- I have two books. Is it possible to find the half of each of these books? How? (Yes, find the total number of pages and divide by 2; or cut the whole book in half.)
- Will half of one book be the same as half of the other book? Why or why not? (No, because one book is larger than the other, so half of that book will be larger than half of the smaller book.)
Use other classroom examples of different-sized similar objects, such as a large sheet of paper and a small sheet, the teacher desk and a student desk, etc.
Then, show two different circle pieces, such as the pink piece and the orange piece, and ask similar questions:
- Which one of these pieces can we call a unit whole? (Both of them.)
- Is it possible to find a half of this pink piece? This orange piece? (Yes, both could be divided in half.)
- What piece is half of the pink piece? (a yellow piece)
- What piece is half of the orange piece? (an aqua piece)
Assign Questions 11–24 to student pairs.

This dialog is adapted from a conversation between a teacher and a group of students who are working on Question 14. Jerome follows the directions for Question 14A and covers a pink piece with an aqua and an orange.
Jerome: I am confused about this part. [Jerome underlines the question, “Do these two pieces divide the pink piece into two halves? Why or why not?]
Teacher: You did what they asked. You covered the pink piece with an aqua and an orange. Let me ask you a question. [She pulls out the pink piece.] What is half of the pink piece? [Jerome covers half of the pink piece with a yellow.] Right. Do the orange and aqua each look like half of the pink piece?
Jerome: No.
Teacher: Why not? What's the problem? [Puts two yellows together, so that Jerome is looking at an aqua and orange together, two yellows, and the pink piece. See Figure 2.]
Teacher: What is the difference between the two yellow pieces and the aqua and orange together?
Jerome: They are the same size.
Teacher: Which are the same size?
Jerome: The two yellow pieces.
Teacher: Okay. Would you agree that each yellow is half of the pink piece? [Points to the two yellows and the pink. Jerome nods in agreement.] So, what about the orange and aqua? Is each piece half of the pink piece?
Jerome: No.
Teacher: Why not?
Jerome: They are not the same size.
Teacher: Okay. You've got it.
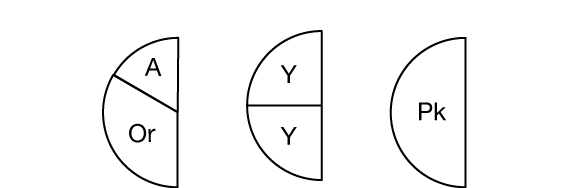
The group turns their attention to Question 14B. As directed, they cover a red circle with a pink and two yellows.
Teacher: Do these three pieces divide the circle into thirds?
Ana: No, because the pieces together do not make thirds.
Teacher: How do you know that?
Ana: Because that [points to the pink] is one-half and that [points to the two yellows] is one-fourth and one-fourth. They are not the same.
Teacher: [Turns to the other students.] What is key in that explanation?
Frank: The pink piece is taking up too much of the space. They are not equal.

Use Check-In: Questions 14–19 in the Student Guide to assess student progress on the following:
- Representing fractions using circle pieces [E1].
- Using words and numbers to name fractions [E2].
- Recognizing that the same fractional parts of different-sized unit wholes are not equal [E3].
- Identifying the unit whole when given a fractional part of a whole [E4].
Targeted practice for these skills and concepts is in the Lesson 7 Workshop.
Notice that Questions 20–24 introduce the green and purple pieces that are one-fifth and one-tenth of the red circle. It may be helpful if the students put away all but the red, pink, green, and purple pieces for these questions.
As students work through the questions, circulate and ask scaffolding questions. Probe student thinking with prompts such as those in the sample dialog for Question 14.
Encourage students to continue using their circle pieces even after they become comfortable visualizing them. As students begin to think more abstractly about fractions, it still pays off to be able to see the actual pieces, their relative sizes, and the number of pieces of one color that fit on another. Using the circle pieces is particularly useful as students work with different-sized unit wholes and try to find the whole when given fractional parts. Ask students to justify their answers to questions using the circle pieces.
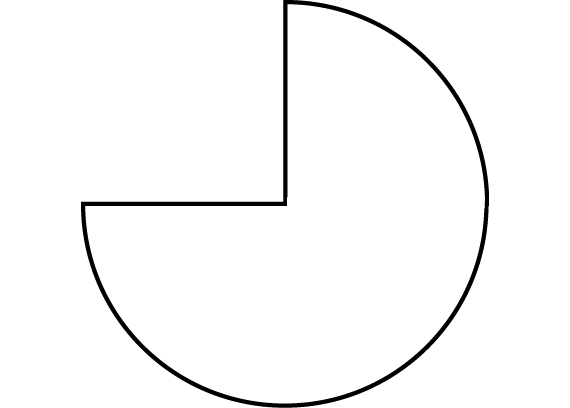
Use prompts similar to the following questions, which pertain to Question 17 and Figure 3.
- What is different about this shape as the unit whole and the red circle as the unit whole? (The red circle is larger; this shape looks like the red circle with one piece taken out of it.)
- If I took half of this shape and a half of the red circle, would the halves be the same size? Why or why not? (No, they would not be the same size, because they are each half of a different-sized whole, i.e., the wholes are not the same size.)
- How many yellow pieces can you fit into this unit whole? (3 yellows)
- What is your numerator when you have one yellow piece? (1)
- What will your denominator be? (3)
- One yellow piece is what fraction of the whole shape? (1/3)
- [Q# 17F] Can you cover the whole shape with just pink pieces without going outside the shape? (no)
- What can you do to figure out what fraction of the shape 1 pink piece is?
- Is there another color that you can use to cover as much as 1 pink piece would cover? (yes)
- Which other color can you use? (yellow)
- How many of those pieces cover 1 pink piece? (2 yellows cover 1 pink piece.)
- How many yellows cover the whole shape? (3 yellows cover the whole shape.)
- The pink piece is what fraction of the whole shape? (The same as 2 yellows, 2/3.)
You may assign Questions 1–6 in the Homework section after Part 2.