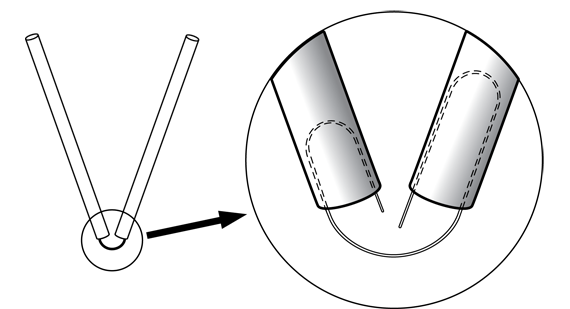Lesson 1

Investigating Angles

Est. Class Sessions: 2

Developing the Lesson

Part 2. Constructing Angles

Make Angles with Straws. Students work in pairs for this activity. Distribute 10 drinking straws and 5 paper clips or pieces of chenille stems to each student. Demonstrate to students how to assemble an angle using two straws as shown in Figure 1. If needed, show students how to bend the ends of the paper clip outward to keep the straws securely attached.

Once students have connected five pairs of straws and paper clips, have them form five different angles. Allow students to play with the straw angles by bending them at the paper clip connection. It is important to help students develop the imagery of angles as “opening” from two overlaid segments fixed at a corner. Demonstrate these images by opening an angle with your straws from 0°, and by bending, or closing, an angle from 180°.

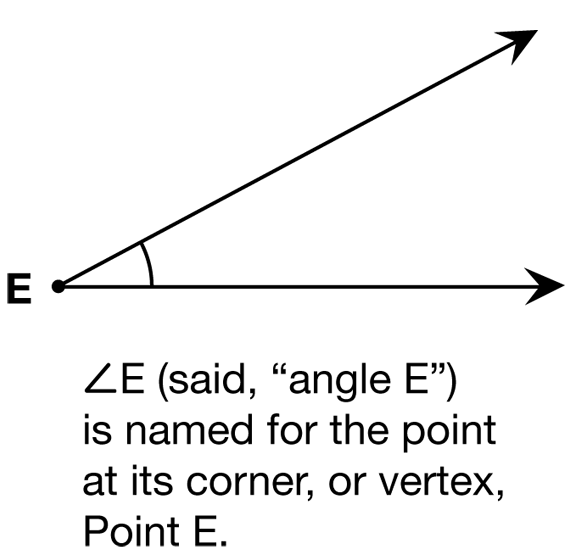
Ask students to construct their five angles to range from small angles (close to 0°) to large angles (close to 180°). Have students sketch and name each angle by labeling the corner (or vertex) point with a capital letter as shown in Figure 2.

Ask the following questions, allowing time after each question for students to discuss their angles with a partner:

X
  • Pick one of your angles. How would you describe it to someone who was not able to see it (as if you were talking to a person on the telephone)? (Possible response: My angle is smaller than a square corner and the sides are very close together.)
  • What do all of your angles have in common? (They have 2 sides that meet at one point.)
  • How are your angles different from each other? (They are different sizes.)
  • What does a small angle look like? Describe it. What makes it small? (It looks sharp. The sides are closer together.)
  • What does a large angle look like? Describe it. What makes it large? (Its opening is wider. Its sides are further apart.)
  • Put all ten of your angles in order from smallest to largest. Do you both agree with the order you decided on?
X

Connect two straws together to make longer sides for the angle by making a small lengthwise fold along the end of one straw and inserting the end into the other straw.

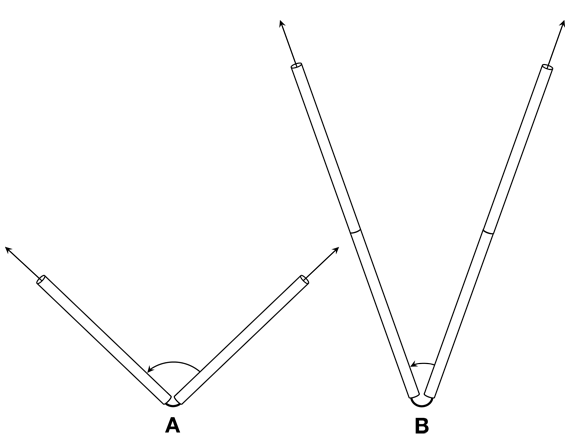
The Meaning of Angle Size. Some students tend to confuse angle size for the size of the segments or rays that intersect to form the angle. Start a class discussion about this by constructing two angles with straws and paper clips as shown in Figure 3. For one angle (∠A), use only one straw per line segment. For the other angle (∠B), use two straws per line segment, but make sure to bend the paper clips so that the angle size of ∠B is smaller than that of ∠A.

Show both angles to the class.

Ask:

X
  • Which angle is larger? (∠A is larger, although some students may think that ∠B is larger since it has longer line segments.)
  • How do you know? (∠A is wider; it shows more turning from the position of one straw to the other.)

If students are still having difficulty distinguishing angle size from segment size, exaggerate the effect by making ∠A very wide and ∠B very narrow. Demonstrate the angle sizes by physically turning the straws from 0° to the final angle positions. You may also draw angles on the board to show how a very sharp angle with long segments is smaller than a very wide angle with short segments because it shows less turning.

X

There will be an opportunity to introduce the terminology of acute angles and obtuse angles at the end of the lesson. These terms are intentionally omitted as part of this activity so that students may explore angle concepts before adopting the formal definitions.

Student Guide:
Student Activity Book:
Master:
Assembling an angle from drinking straws and a paper clip
X
+
Example of drawing and naming an angle
X
+
Comparing angle sizes
X
+