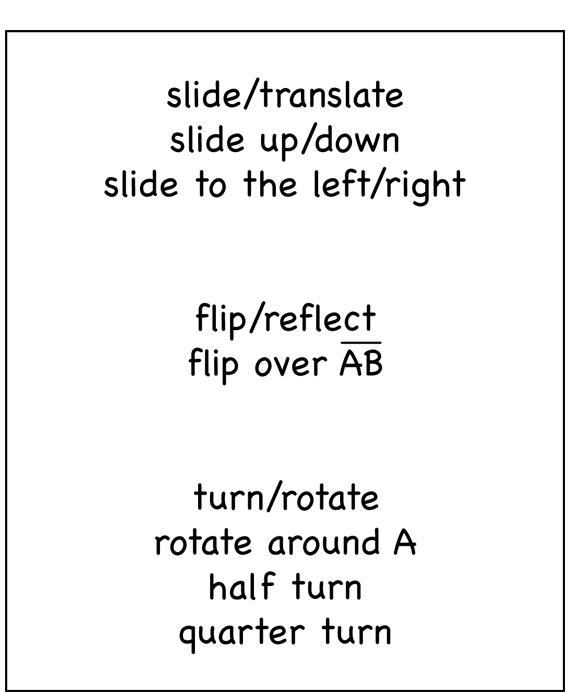
Slides, Flips, and Turns

Est. Class Sessions: 2

Developing the Lesson

Part 2. Slides, Flips, and Turns of Shapes

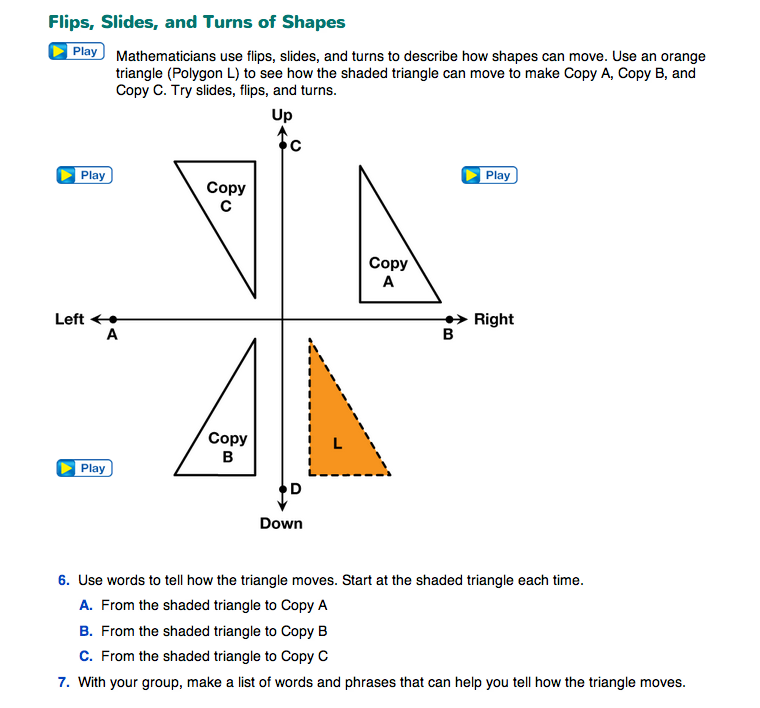
Transform the Triangle. Refer students to Question 6 in the Student Guide. In the introduction to this problem, students describe how a triangle moves to a copy of that triangle. The shapes on the page are based on the orange triangle from the Power Polygons™. Encourage students to manipulate the orange triangle to answer the questions. Copy A is made by sliding the triangle from its original place. Copy B is made by flipping the triangle over 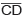. The orange triangle can move different ways to make Copy C. 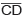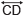The orange triangle can be flipped over and then over , or the orange triangle can be turned around the point of intersection of and 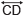. Have students use the orange triangle to show both of these transformations or movements.

Assign Questions 6–8. In Question 7, small groups are asked to generate a list of words and phrases that can be used to describe how the triangle moves. Once groups have had a chance to generate a list, collect the words and phrases on the chart paper you prepared to add to the Geometry Word Chart. Leave enough room between words and phrases to add pictures later. See Figure 3.

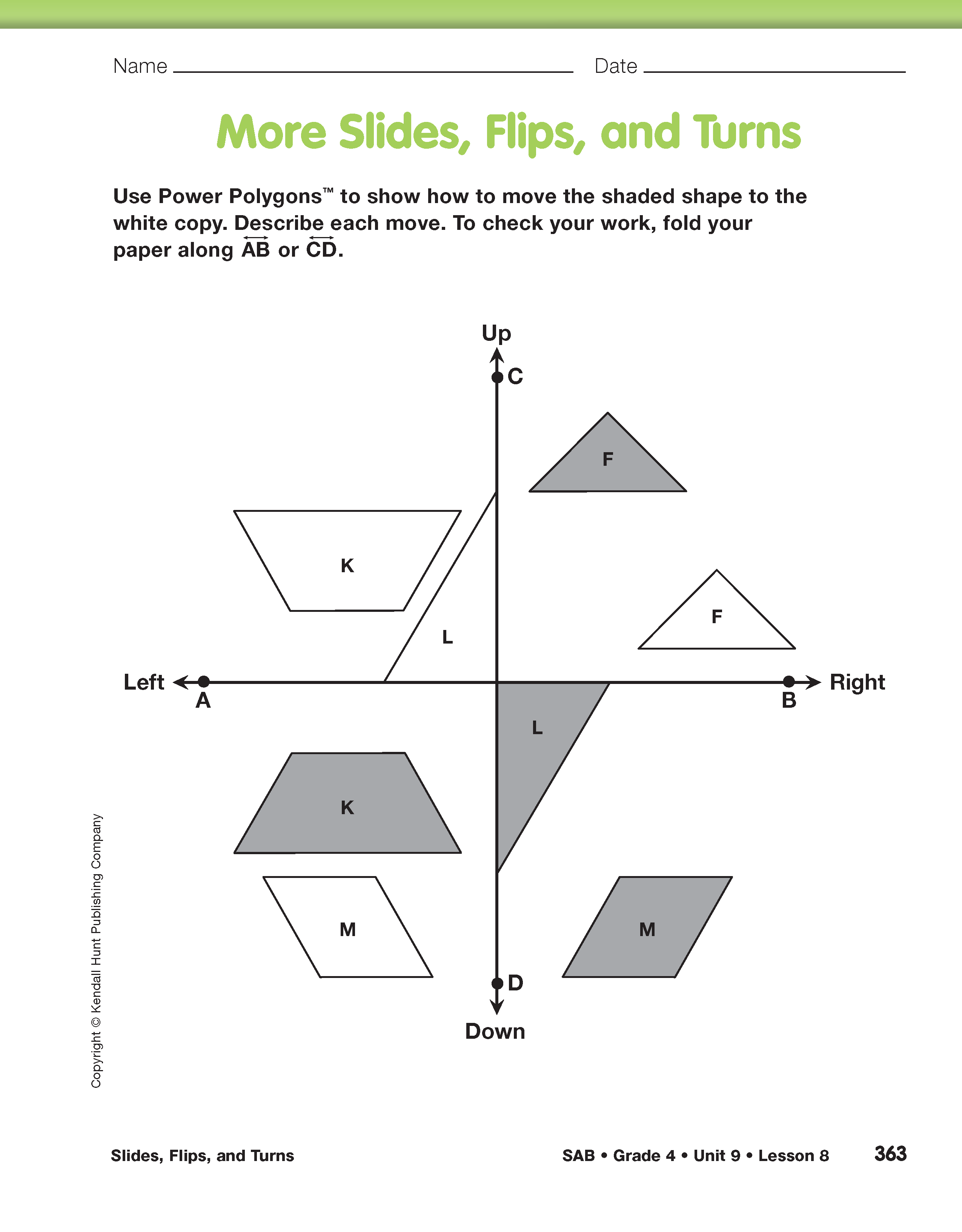
Transform the Polygons. Ask students to remove the More Slides, Flips, and Turns page from the Student Activity Book. Students use the Power Polygons™ to show how a shaded shape moves to a white copy. As students are describing the shapes' movements, ask them to use some of the words and phrases they listed on the Geometry Word Chart previously.

Ask:

Which shapes are flipped? (Trapezoid K is flipped over and Rhombus M is flipped over .)
How can you check if it is really flipped over the line? (Fold on the line. If the shape is really flipped over that line the shapes will line up exactly. Each shape will be the same distance from the line and the shapes will be a mirror image.)
Which shapes are slides? (Triangle F is a slide.)
How do you know? (The shape does not change orientation, it is simply in a different place.)
Describe the slide. (Triangle F slid down and to the right to match the white copy.)
How is Triangle L moved? (Triangle L can turn a half turn or flip once over and then over to make the white copy.)

Introduce Congruence.

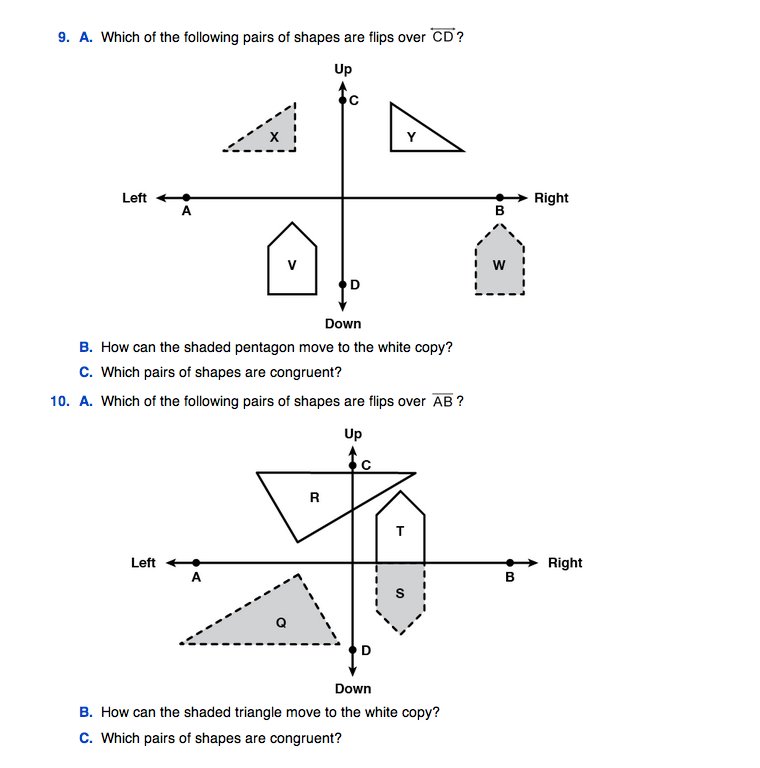
Referring to the pairs of shapes on the More Slides, Flips, and Turns page, ask:

What is the same about each pair of shapes? (The shape is the same.)
What is different about each pair of shapes? (Where the shape is or how the shape is turned)

Tell students these pairs of shapes are called congruent shapes. Congruent shapes are the same size and shape. Shapes are congruent if you can move one shape so it fits on top of the other and they match exactly.

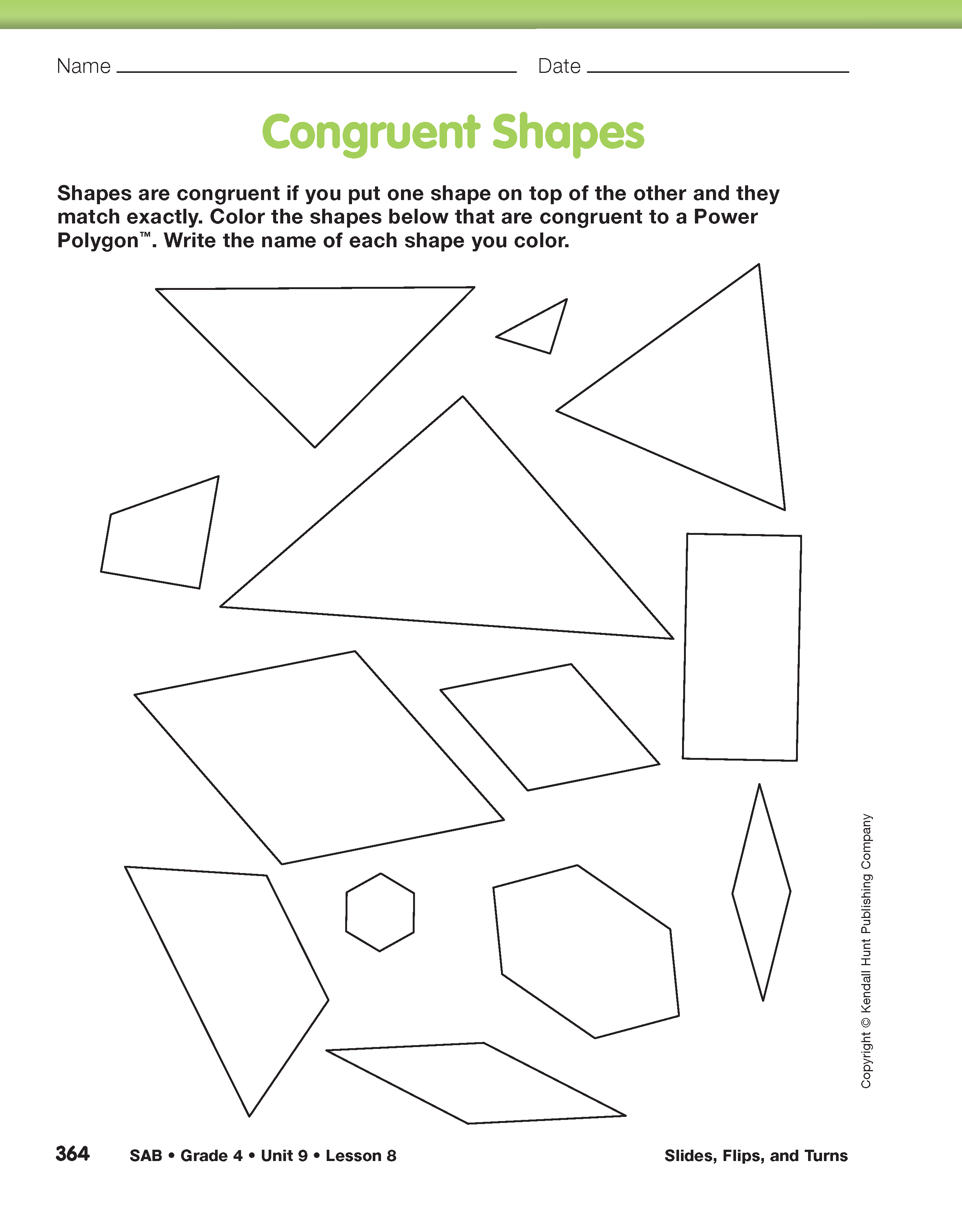
To help students identify congruent shapes, assign the Congruent Shapes page in the Student Activity Book. Students identify shapes that are congruent to the Power Polygons™.

After students have colored and labeled the congruent shapes, ask:

Why are the other shapes not congruent to the Power Polygons? (Possible responses: The shape does not match any of the polygons exactly; or the triangle is the right shape but it is too small, so it is not congruent.)

To further explore transformations and identify congruent shapes, direct students' attention to the Congruence section in the Student Guide and discuss Questions 9–10. Questions 9 and 10 explore more than one way to move a shape to a copy of that shape. For example, in Question 9, Pentagon V and W are not flips over 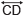. Copy V can be made by sliding W or by flipping W over 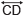and sliding to the right.

In Question 10, Triangle Q can be rotated to make Copy R. Triangle Q can also be flipped over , then flipped over 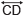, then slid to the left to make Copy R.