Workshop: Angles and Lines

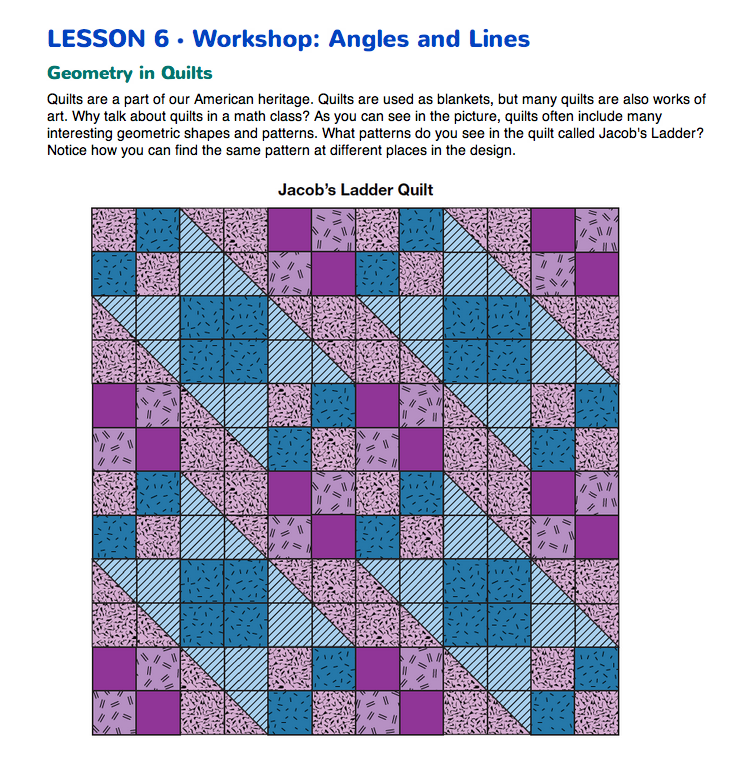
Begin class by displaying the Jacob's Ladder Quilt on the Angles and Lines pages in the Student Guide. Discuss for a few minutes the history of quilt-making. See the Content Note. Ask students what they know of quilts, whether they have one at home, or if they know anyone who makes quilts.

Some quilts use geometric patterns for their designs. Ask students to take a close look at the Jacob's Ladder Quilt.

Remind students of the measures of the angles of the square.

Students may notice that the triangle has one right angle and reason that the two acute angles must both be 45° because the two angles cut a right angle exactly in half. They may also remember that the sum of the angle measures of a triangle is 180° and use that information to reason that the acute angles must be 45°. (180 − 90 = 90; 90 ÷ 2 = 45.)

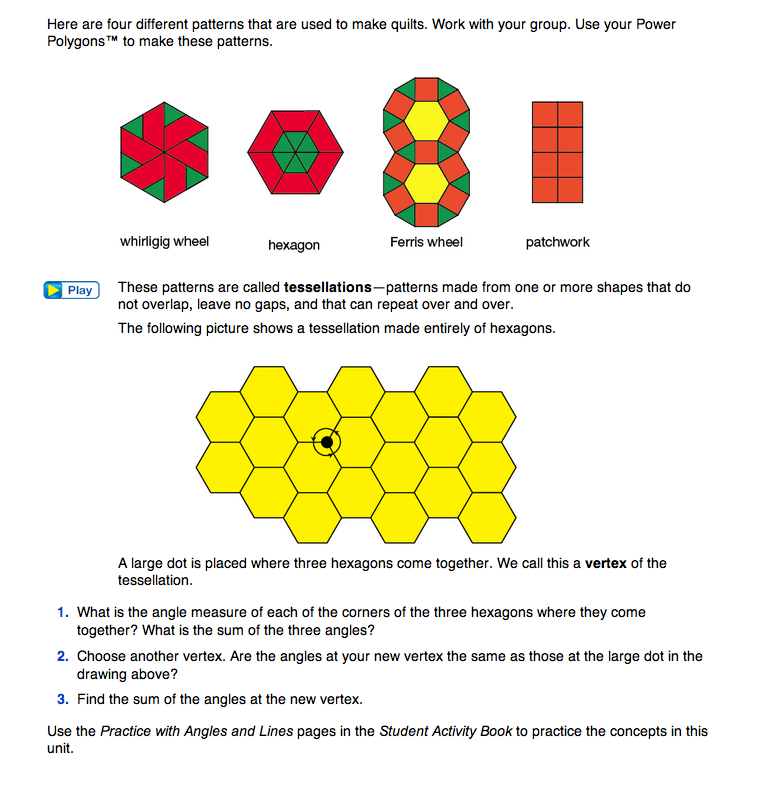
Point out to students that all the pieces fit together exactly, leaving no gaps or overlaps. Refer students to and display the four quilt patterns after the Jacob's Ladder Quilt in the Student Guide. Ask students to look at the four quilt patterns and tell them these patterns are tessellations. They are created when a shape is repeated over and over again covering a surface without leaving gaps. Ask students to work with partners to complete Questions 1–3 to investigate the angles in tessellations. For shapes to meet at a point, or vertex, and leave no gaps, the angle measures at the vertex must total 360 degrees.